TOPIC 1: INTRODUCTION TO PHYSICS








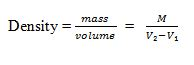

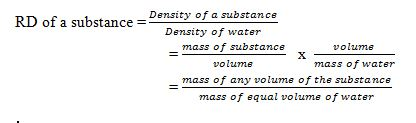

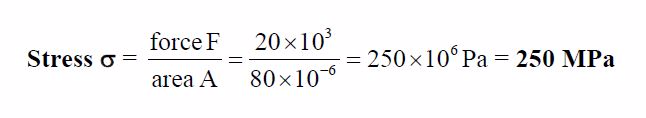

TOPIC 5: ARCHIMEDES' PRINCIPLE AND LAW OF FLOTATION


TOPIC 7: PRESSURE



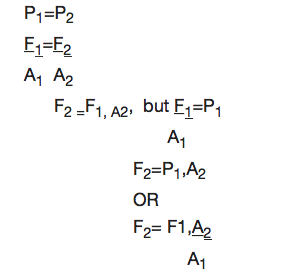
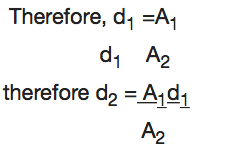
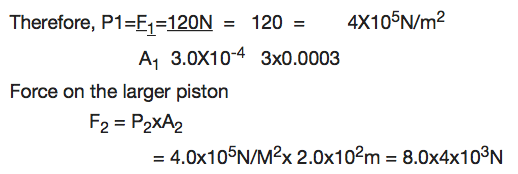


 TOPIC 8: WORK ENERGY AND POWER
TOPIC 8: WORK ENERGY AND POWER


 TOPIC 9: LIGHT
TOPIC 9: LIGHT



Physics
is often described as the study of matter and energy. It is concerned
with how matter and energy relate to each other, and how they affect
each other over time and through space. Physicists ask the fundamental
questions how did the universe begin? how and of what is it made? how
does it change? what rules govern its behavior?
Concepts of Physics
The Concepts of Physics
Explain the concepts of physics
Physics is described as the general scientific study of nature that aims to understand the behavior of the universe.
Physics, therefore tries to explain how things happen around us or why
they happen the way they do. For example, how a car moves from one point
to another? Why unlike poles of magnets attract? Why does a mango fall?
How is a rainbow formed? And so on.
Physics
as a subject uses concepts like force, energy, mass, power among others
to explain different phenomena. Energy, for example, may take the form
of motion, light or electricity. In so doing, students of Physics get to
learn more about matter and energy and how they interact with each
other.
Therefore, Physics is the study of the relationship between matter and energy.
The people who study and work professionally in this field are called physicists.
Physics
is an experimental science subject, which creates theories that are
tested against observations of the natural world. Theories are used to
explain why things behave the way they do. This is why physicists always
insist on accurate measurements and a record of results during each experiment.
Once
these results are in precise mathematical forms, technologists make use
of such data when designing devices such as radios, televisions, mobile
phones, computers, and artificial satellites. All these things are for
the betterment of human life.
The Relationship between Physics and other Subjects
Establish the relationship between physics and other subjects
Knowledge
of physics is also applied in other subjects. The principles of
electrostatics and particulate nature, for example, are used to explain
reactions in chemistry. The tools, implements and farm machinery learnt
in agriculture as well as the process of dating historical remains are
applications of physics.
Physics
provides the basis for biology. Without space, matter, energy and time
-- components that make up the universe -- living organisms would not
exist. Physicist Richard Feynman said that everything on earth is made
of atoms, basic units of matter, that constantly move. Since biology has
its foundation in physics, it applies physical natural laws to the
study of living organisms, according to Muskegon Community College. For
instance, physics helps explain how bats use sound waves to navigate in
the dark and how wings give insects the ability to move through the air.
The American Physical Society shares that many flowers arrange their
seeds or petals in a Fibonacci-like sequence to maximize exposure to
light and nutrients. In some cases, biology helps prove physical laws
and theories. Feynman states that biology helped scientists come up with
the law of conservation of energy.
Importance of Studying Physics
State the importance of studying physics
Why Study Physics?
- Physics helps us tounderstand how the world around us works, from can openers, light bulbs and cell phones to muscles, lungs and brains; from paints, piccolos and pirouettes to cameras, cars and cathedrals; from earthquakes, tsunamis and hurricanes to quarks, DNA and black holes. From the prosaic . . . to the profound . . . to the poetic. . .
- Physics helps us toorganize the universe.It deals with fundamentals, and helps us tosee the connectionsbetween seemly disparate phenomena.Physics gives us powerful tools to help us toexpress our creativity, to see the world in new ways and then to change it.
- Physics providesquantitative and analytic skillsneeded foranalyzing data and solving problemsin the sciences, engineering and medicine, as well as in economics, finance, management, law and public policy.
- Physics is the basis for most modern technology, and for the tools and instruments used in scientific, engineering and medical research and development. Manufacturing is dominated by physics-based technology.
- Physics helps you to help others.Doctors that don’t understand physics can be dangerous. Medicine without physics technology would be barbaric. Schools without qualified physics teachers cut their students off from a host of well-respected, well paying careers.
Applications of Physics in Real Life
Discoveries in Physics have led to various inventions that influence our lives.
- At home;All tools and machinery that we use in our homes to make work easier are made in accordance with the laws of Physics. They include crowbars, hammers, door handles, cutlery, hinges, car jack, pulleys, tillage implements, and so many other things. For example, it would be difficult for us to try to lift a car so as to change its tyres. However, using a car jack makes this task easy. Electrical appliances such as cooker, iron, heater, electric lamps, washing machine and so on are a result of the application of the knowledge of physics.
- Medical field; A variety of medical processes and machinery rely on the application of the knowledge of Physics. Some of these machines are used in diagnosis and treatment of various ailments as well as sustaining the lives of patients through certain forms of therapy. Examples of machines used in hospitals include laser, x-ray, incubators, ultrasound and infrared machines. The knowledge used in handling and even actual use of these machines is based on the knowledge and skills acquired in Physics.
- Source of energy; Some processes and machines help us to obtain energy for our daily use. These machines make use of various laws of physics to give us different forms of energy. For example, batteries and generators provide electrical energy that is readily used in radios and televisions. A car battery provides energy needed to drive a car. When devices like bulbs are connected to these sources, they provide light energy for daily use.
- Transport; Application of laws of Physics governing concepts such as friction and frictional forces ensures that human beings and animals can walk, run and stop without falling over. Vessels used in transportation such as cars, ships, aeroplanes and trains are also able to move, brake and stop when necessary. This is all because the laws related to friction; flotation and balance are observed and applied accordingly. When these laws are disobeyed, ships sink and trains derail.
- Communication; Devices used in communication systems such as telephones, modems for accessing the internet and television, use cables, telecommunication transmitters and receivers to relay information. The knowledge of Physics is essential, because these instruments make use of fiber optics and radio waves in order to relay messages. Newspapers, letters, electronic mails (email), fax massages from fax machines and short messages (SMS) through mobile phones are reliable means of communication. All these things require the knowledge of Physics.
- Entertainment; Physics enables people to enjoy a variety of leisure activities as is evident in photography, digital appliances, exercise machines and other sport equipment.
- Industry; Physicists have been able to come up with tools and process that have resulted in advanced technological equipment and new discoveries. These include highly accurate skills such as computer assembly and computer programs used in design; and instruments like binoculars and telescopes; the ability to land on the moon and measure the temperature of bodies are a few examples.
- In schools;The instruments and apparatus used in school laboratories are made through the application of the knowledge and skill acquired in a Physics class. These apparatus have to meet certain specifications or standards that are universally accepted.
Physics in Daily Life
Apply physics in daily life
Driving
a car requires a basic understanding of the principles of physics,
whether the driver is conscious of them or not. For instance, the
balance of a car changes as the car accelerates, decelerates or turns.
To keep the car on the road, the driver must anticipate these forces
acting on the car and make adjustments.
Having
an understanding of physics may also help an individual to lift
something heavy. For example, by using a simple machine such as an
inclined plane or a lever, an individual can make the load easier to
lift. Physicists study simple machines extensively and use the lessons
they learn to make increasingly efficient tools.
To
stay warm in the winter, people up turn the heat up or use the
principles of physics to understand how to make better use of the heat
that is already in their homes. Heat flows via conductive, radiant and
convective means; understanding the differences between the three forms
will allow an individual to insulate a house better. For example, by
using ceiling fans intelligently, someone can make the convective heat
flow in a house work to their advantage.
TOPIC 2: INTRODUCTION TO LABORATORY PRACTICE
A Laboratory is a room or a building designed specifically for carrying out experiments.
Laboratory Rules and Safety Guidelines
Rules in a Physics Laboratory
State rules in physics laboratory
While
working in the lab, individuals are advised to adhere to specific lab
rules to ensure their safety, that of other people working in the lab
and the safety of the apparatus and chemicals stored in the lab.
Rules in the physics lab
- You should only enter the lab in the presence of a lab attendant.
- Keep all exits and entrances to and from the lab clear of obstructions.
- Don’t eat or drink in the lab.
- Solid objects should not be thrown into the sink or water ways.
- Don’t use any lab container for drinking or storing food.
- Make sure that you wash your hands with soap before you leave the lab.
- The cover or stopper of a particular chemical must be replaced immediately after use.
- After experiments, clean your bench and leave it dry and well arranged.
- Never quarrel or fight in the lab.
- Never use ungloved hands to hold hot objects
Safety Measures in the Physics Laboratory
Explain the safety measures in physics laboratory
Safety measures in physics lab
- All experiments which produce poisonous fumes must be conducted in the fume chamber.
- Lab floor should not be polished to avoid slipperiness.
- Lab should have large windows and doors should be opened outwards.
- Ensure that the fire extinguisher is fixed to an appropriate place ready to be accessed in case of fire.
- In a multi storey building, a physics lab should be in the lowest floor.
Using First Aid Kit to Render First Aid
Use the First Aid Kit to render first aid
First aid
is the immediate care given to accident victims or an injured person
before he/she is taken to the hospital for further medical treatment. It
reduces pain and it helps to bring hope and encouragement to accident
victims.
A First Aid Kit is
a small box which is placed in a safe and accessible place in the lab
and is used for the storage of instruments and chemicals for first aid.
Contents of a first aid kit and their uses
| Contents | Uses |
| 1. Pair of scissors | To cut adhesive tapes, bandages and gauze |
| 2. Rolls of adhesive tape | To hold firmly into wounds bandages, gauze and cotton wool |
| 3. Bandages and cotton wool | To clean and cover wounds |
| 4. Sterilised new razor blades | Used when treating new or old wound |
| 5. Sterilised wound | To clean and cover wounds |
| 6. Safety pin | To tighten clip bandages |
| 7. One jar of petroleum jelly | To apply on burns |
| 8. Iodine tincture | To clean fresh cuts and bruises |
| 9. Soap | To wash hands and wounds |
| 10. Antibiotic solution. | To clean wounds |
Warning Signs
Identify warning signs
Warning
signs are signs on the containers of chemicals as warnings about the
danger of the contained chemical. There are six warning signs.
Explosives
These
are substances can explode. An explosion is a forceful rapid reaction
which involves the throwing off particles at high speeds.

The sign means that it is dangerous to keep explosives in glass containers.
Oxidant
This is a chemical/substance that helps a burning substance to burn faster.

Oxidising
agent makes a small fire to become bigger. Heating a mixture of an
organic material with an oxidising agent may cause explosion. Eg;
heating potassium permanganate with saw dust.
Flammable
These substances catch fire easily. They should not be kept near open flames. If heated,anelectric heater should be used.

Corrosive
These substances burn skin as well can corrode floors and desktops.

If
by accident, a corrosive substance comes into contact with your skin,
go to the sink and wash your skin with a lot of water. Examples;
Concentrated mineral acids like HCl and HNO3 II. Concentrated alkalis
like NaOH and KOH
Toxic
These
are very poisonous and can cause death immediately after use or after a
few days. They should not be allowed to come into contact with you.

-If you come into contact with such a chemical accidentally, wash it away with a lot of water.
Harmful/irritant
Harmful
- these substances may cause illness or endanger your health. They
won’t kill instantly but they are lethal. Irritating substances- cause
pain in eyes or on your skin and can endanger your health if you are in
contact with them doe too long.

They should be handled according to the stipulated instructions.
Warning Signs in Daily Life
Use warning signs in daily life
Activity 1
Use warning signs in daily life
Basic Principles of Science Investigation
The Concept of Scientific Investigation
Explain the concept of scientific investigation
Scientific investigation refers to the step-by-step procedures and methods employed in carrying out a scientific research.
Steps of Scientific Investigation
Identify the steps of scientific investigation
There are six different scientific step:
- Identification of a problem:This is the first step where a problem is recognised. It provides explanations to the day-to-day questions which we come across in our lives Eg; It is observed that despite adequate feeding, vaccination, treatment and spacious rooms, hens lay fewer eggs each day. What is the cause of the fewer number of eggs?
- Formulation of hypothesis: Hypothesis is an intelligent guess or a tentative explanation for the observation made. Using the example of a few eggs laid by hens per day the hypothesis could be; a smaller number of eggs are laid because of unusual high temperature in the room. In order to prove this hypothesis, an experiment have to be carried out.
- Experimentation: The experimentinvolvesa series of investigations intended to discover relationships or certain facts that may lead to the acceptance, rejection or modification of a hypothesis. The first step in experiment is to construct a plan of investigation.
- Observation and data recording:After setting up the experiment, a researcher makes careful observation using their sense organs and records all events that considers relevant.
- Interpretation of data:Once a researcher has collected data, he should try to explain the meaning of the experiment. This is an attempt to interpret the data. The data may be presented in a form of a table, bar chart, histogram or even graph.
- Conclusion:This is either confirmation or rejection of hypothesis.
A Theory
is a set scientific assumptions consistent with one another and
supported by evidence but not fully proved (is a hypothesis with more
evidence).
Fact is a proved theory supported by evidence
Hypothesis →Theory →Fact
Scientifc Investigation Methods in Solving Problems
Use the scientific investigation methods in solving problems
Activity 2
Use the scientific investigation methods in solving problems
TOPIC 3: MEASUREMENT
Concepts of Measurement
The Concepts of Measurement
Explain the concepts of measurement
Measurement is the process of assigning numbers to observations or events.
Importance of Measurement in Real Life
State the importance of measurement in real life
Measurements
are so often taken for granted, we sometimes do not appreciate the
grand importance measurements play in our lives. On a baseline level,
measurements fall into the categories of weight, area, volume, length
and even temperature. While we look at these various categories as stoic
forms of mathematical measurements, a closer examination of things we
do in everyday life reveals their clear importance.
Taking proper medicine.
If you are ill (whether a serious or minor illness) you need to take
your medicine and take it in the proper amount. If you take too little
or too much then you are not going to get the proper benefit from it.
Cooking properly.
Cooking of all forms is based on proper attention to measurement. Can
you bake a chicken at 600 degrees? Well, you can but the results would
be pretty catastrophic! Could you may a cup of tea by dipping a tea bag
into a teaspoon of warm water?
Purchasing clothes.
Now, how important could measurements be when selecting clothes? After
all, to look good in clothing the main thing you need to pay attention
to is style, right? Well, if you weigh 200lbs you aren't going to look
stylish in an extra small shirt. Clothing is all based on size and
proper fitting which are, of course, variants of measurement.
Playing sports.
The importance of measurements may not necessarily reveal itself when
you play sports but it is there in a big way. If you want to throw a
runner out at first or make a 30 yard touchdown pass then you really
need to be accurate and comes from a clear sense of depth and distance.
Making estimations.
If you have to be at school at 9am what time would you have to leave in
the morning if you are at your friend's house. Often we do not have an
exact answer so we need to make an estimation which is essentially a
guess of measurement.
Keeping yourself warm or cool.
If the temperature outside dips or increases you have to make an
adjustment on your thermostat in order to remain at a safe and healthy
temperature. Understanding the measurement scale of a thermostat is
critical in this regard or else you may find yourself feeling rather
uncomfortable.
Understanding weight.
Is that object too heavy to pick up by yourself or do you need to use
something to lift it? Some may think this is not important but it is
pretty easy to hurt yourself if you lift objects that are too heavy.
Proper use of capacity.
Just how many clothes can you fit in a dresser or closet without it
becoming too crammed? Without a clear concept of capacity you might find
yourself pouring an entire half gallon of orange juice into a small
glass!
Telling time.
The ability to tell time is all based on measurement principles.
Whether you are using a digital clock or an hourglass these devices
measure the passage of time. Now, imagine how chaotic the world would be
if if was impossible to measure the passage of time.
Transportation.
How much weight is too much for a plane to take off or a car to move
efficiently? How much fuel is needed to reach a certain point and how
long will it take to get somewhere? Yes, measurements play a significant
part in transportation.
Structure.
This was saved for last because it is the common theme that is found in
all the multitude of reasons for the importance of measurements.
Measurements provide structure and remove the chaos that would result
without any congruent method of understanding weight, mass, temperature,
etc.
Basic Fundamental Quantities
A Fundamental Quantity
Define a fundamental quantity
Physical quantity:Is any character which can be measured by an instrument.
A Unitis the standard which is used to explain measurement of a body.Eg; kilogram, metre, second etc.
Fundamental quantitiesare numbers that we need to describe the world around us, which we cannot express in terms of "simpler," more basicquantities. Here is an example: My weight is not afundamental quantity, because it depends on how much stuff makes up my body.
Three Basic Fundamental Quantities of Measurement
Mention three basic fundamental quantities of measurement
Basic fundamental quantitiesare physical quantities from which other physical quantities are derived from. This includes three quantities namely mass, length and time.
The S.I Unit of Fundamental Quantity
State the S.I unit of fundamental quantities
SI unit (International
system of units): Is the system of units which is used internationally
to measure three basic physical quantities.
SI units of fundamental quantities
| Basic physical quantity | SI unit |
| Mass | Kilogram (kg) |
| Length | Meter (m) |
| Time | Second(s |
Metric system
Is
an international system which is a decimal based system, consequently,
conversions from one unit to another within the metric system can
accomplished by multiplying or dividing by ten or power of ten.
Note:
With the exception of temperature, amount of substance and luminous
intensity international other units of measurement that are smaller or
larger than the most commonly used units are expressed by attaching a
prefix to the most commonly used units.
More than 1 unit
- Giga(G) = 1,000,000,000 (10ˆ9)
- Mega(M) = 1,000,000 (10ˆ6)
- Kilo(K) = 1,000(10ˆ3)
- Hector (h) = 100(10ˆ2)
- Decca(da) = 10(10ˆ1)1
Less than 1 unit
- Deci (d) = 1/10 (10ˆ-1)
- Cent (c) = 1/100(10ˆ-2)
- Mill (m) = 1/1000 (10ˆ-3)
- Micro(μ) = 1/1,000,000(10ˆ-6)
Appropriate Instruments for Measuring Fundamental Quantities
Use appropriate instruments for measuring fundamental quantities
Length, l
Length is the distance between two points, objects or space.The SI unit of length is meter(m). Other commonly used units are kilometer(km) and centimeter(cm).
1km = 1000m
1m = 100cm
The instrument used to measure length is the metre rule.
How to read the metre rule:Owing
to the thickness of the wood,the eye must always be placed vertically
above the mark being read, in order to avoid errors due to parallax.
Measuring of length (diameter) of small objects.
The diameter of small objects is measured by using two instruments:
- Vernier caliper
- Micrometer screw gauge
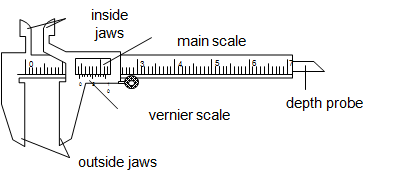
A venier caliper is the instrument used to measure length to the accuracy of 0.01cm.It is used to measure lengths to the range of 1.0cm to about 12.0cm.The figure below describe the structure of vernier caliper.

The
main scale is graduated in centimeter (cm) while the vernier scale is
graduated in millimeter (mm).The vernier scale is a short scale 9mm long
divided into 10 equal parts, so that the difference in length between a
vernier division and the main scale division is 0.1mm or 0.01cm.
The
inside jaws are used to measure the inside diameter while the outside
jaws are used to measure outside diameter.The vernier slides over the
main scale.
How to read
- The main scale reading is recorded. This is the reading which precedes the zero mark of the vernier scale.
- The vernier scale reading is recorded by reading the mark on it which coincide with a mark on the main scale (i.e. vernier scale reading x 0.01cm).
- The summation of these two readings is the length of the object measured.
A micrometer screw gauge:Is an instrument used to measure length to the accuracy of 0.001cm(0.01mm).It
is used to measure the diameters of wires and ball bearings. It can
measure small lengths up to about 2.5cm.The diagram below describes the
micrometer screw gauge.

It
consists of a spindle which is fitted with a graduated thimble. The
screwed portion of a spindle is totally enclosed to protect it from
damage.The pitch of the screw is 0.5mm, so that the spindle moves
through o.o5cm for each complete turn.
The
anvil and the spindle grip the measured object between them. The
ratchet prevents the user from using undue pressure. The sleeve is
graduated in mm, each graduation represent one complete turn of the
screw.
How to read a micrometer screw gauge:
- Sleeve reading is recorded. This gives the units and the first two decimal places in mm.
- Thimble reading is then recorded. This gives the third decimal place (thimble reading x 0.001mm).
- The summation of these two readings gives the diameter of the object under measurement.
Precautions when using a micrometer screw gauge.
- Before use,the faces of anvil and spindle should be wiped clean to remove any dirty particle which would give false readings.
- Check and record for zero error then + or –the correction to the final answer.
Mass
Mass of a body is the amount of matter it contains. The SI unit of mass is kilogram(kg). Other commonly used units are gram(g) and tones(t).
1kg = 1000g
1t = 1000kg
The mass of a body doesn’t change from place to place. The instrument used to measure mass is called abeam balance.

Difference between mass and weight:
| Mass | Weight |
| Is the amount of matter contained | Is the force by which the earth pull a body to its centre |
| SI unit is kilogram | SI unit is Newton |
| Doesn’t vary from place to place on the earth’s surface | Varies from place to place on the earth’s surface |
| Measured by beam balance | Measured by a spring balance |
Time
Is the gap between two occasions or events.The SI unit of time second(s). Other units used are minutes (min), hour(h),day etc.
1min = 60s
1h = 3600s
1day = 86400s
The instruments for measuring time are clocks and watches.

Derived quantities
Derived Quantities
Explain derived quantities
Derived quantities are
units which are derived from the fundamental quantities. Examples are
volume, density, power, work, energy, weight, frequency etc.
The S.I Units of Derived Quantities
State the S.I. units of derived quantities
SI units of Derived quantities
| Volume | Cubic meter (mˆ3) |
| Density | Kg/mˆ3 |
| Power | Watts (W) |
| Work | Joules (J) |
| Energy | Joules (J) |
| Weight | Newton (N) |
| Frequency | Hertz (Hz) |
Basic Apparatus/equipment's and their uses
Basic Apparatus/Equipments Used for Measurement
Describe basic apparatus/equipments used for measurement
Volume
Volume
is the amount of space occupied by a substance. The SI unit is cubic
meter (mˆ3).Other units used are cubic centimetre (cmˆ3) and litre(l).
Instruments used to measure the volume of liquids:
Measuring cylinder-used for measuring or pouring out various liquids.

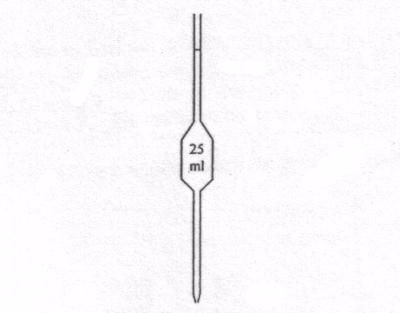
Measuring flask and pipette are used for getting fixed pre-determined volume.

Flask

Pipette
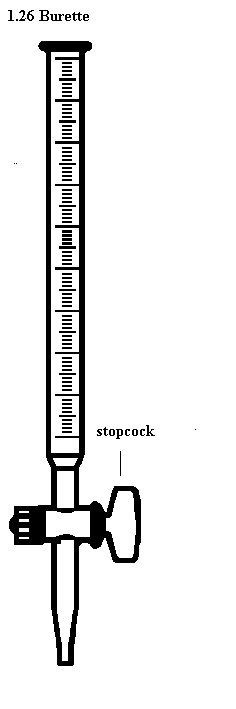
Burette-used to deliver any required volume up to its total capacity.

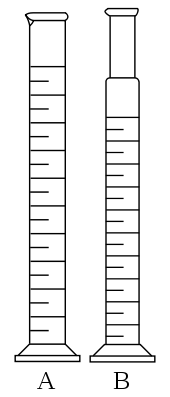
How to read volume measuring instruments(precautions).
Readings
are always taken at the level of the bottom of the meniscus or curved
surface of the liquid. Mercury is an exception as its meniscus curves
downwards.
Care
should be taken to place the eye correctly to avoid parallax errors.
When taking readings, the pipette and burette must be upright and the
cylinder and flask must stand on a horizontal bench otherwise errors may
arise from tilting.
Measuring volume of irregular objects.
The
volume of an irregular solid can be determined by measuring the volume
of water displaced in a measuring cylinder directly or with the aid of
an overflow eureka can.
Activity 1
Experiment
Aim: To measure the volume of an irregular object.
Methods
By using a measuring cylinder directly
Materials and apparatus: Irregular object eg; stone, thread, measuring cylinder, eureka can and water.
Procedures
- Poor a known volume of water in a burette(V1)
- Tie a stone with a thread.
- Immerse the tied stone in water holding the thread and record the volume (V2)
- Make sure the stone is totally immersed in water.
Results
- Volume before introducing solid = V1
- Volume after introducing solid = V2
- Volume of irregular solid(V3) = V2 – V1
By using the eureka can
Procedureto follow:
- Poor water into eureka can up to its spout
- Immerse a well tied stone in water completely
- Collect the overflowed water in the water.
- Use a measuring cylinder to determine the volume of water collected
Observation
- When a stone was introduced in an overflow can, water overflowed to the measuring cylinder.
- The volume of water collected is equal to the volume of irregular object(stone)
Sources of Errors in Measurement
Identify sources of errors in measurement
Error
is the difference between the measured value and the real or actual
value (The difference in reading is known as the error).
There are two types of errors, namely:
- Systematic errors
- Random errors
Systematic errors
Systematic
errors results in the measurement or reading being consistently over
the actual value OR consistently smaller than the actual value.
Sources of systematic errors.
- Zero error: Zero Error is caused if the reading shown is Not zero when the true value is actually zero. This is most probably caused by a flaw in the instrument for example when using a ruler that has lost its zero scale due to wear and tear hence causing an error in the measurement of length.
- Wrong assumptions: For example if you assume that water boils at 100 degree Celsius but actually its boiling point is higher if there are impurities in it. (Pure water boils at 100 degree Celsius).
- Lag of reaction time: For example in a sports day, when measuring a 100 m running time using a stopwatch. The observer may not press the stop button exactly when the foot of the runner touches the finishing line.
- Calibration errors: Instruments that are not properly calibrated could also cause error and this has to be put in consideration when writing a report or when there is an anomaly in reading.
Random errors.
Random
error is caused by the observer who reads the measuring instrument.
Just like the systematic error, there is also positive or negative
error. Positive error is when the reading is bigger than the real value
and negative error is when the reading is smaller than the real value.
Ways of reducing errors
- Taking several readings and then find the average.
- Avoiding parallax error by positioning the instrument (meter rule) properly on the table with the eyes perpendicular to the scale.
- Some instruments can be adjusted to eliminate zero error. For example when using an ammeter, there is an adjuster to set the indicator to zero before making any measurement.
- In the case of a ruler, measurement can be carried out starting from the next clear scale for example if scale 0.0cm is blurred, we can start measuring the length from 2.0cm, of course taking the difference of value in consideration when recording the final reading.
Density and Relative Density
The Concept of Density of a Substance and its S.I Unit
Explain the concept of density of a substance and its S.I unit
The Density of a substance is its mass per unit volume.
The
unit of density is kg/mˆ3. Other unit used is g/cmˆ3.Density of regular
solid object can easily be found by direct and easy measurements.-It
involves measuring the mass and calculating the volume as described in
the experiment below.
The Density of Regular and Irregular Solids
Determine the density of regular and irregular solids
Activity 2
Experiment
Aim;To measure the density of rectangular block.
Material and Apparatus :Ruler, beam balance and rectangular block.
Procedures:Using a beam balance measure mass of the block, m.Measure its length, width and height.
Results
- The mass of the rectangular block is m.
- The volume of the rectangular block will be calculated by multiplying the obtained length, l height, h and width, w.
- Volume, V = l x h x w, But; Density = mass/volume
- The volume of a material can be obtained by using various methods depending on the shape of the material
Activity 3
Experiment.
Aim; To determine density of irregular solid.
Materials and apparatus:Irregular solid like stone, measuring cylinder, beam balance and water.
Procedures
- Obtain the mass of the given object using the beam balance.
- Fill water to the measuring cylinder to the volume V₁.
- Immerse the well tied irregular object totally in the cylinder containing water.
- Record the new volume V₂.
Results
- Volume of irregular object = V₂ - V₁
- Mass obtained = M
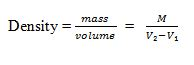
The Density of a Liquid
Determine the density of a liquid
Density of liquids can be determined by using a burrete or a density.
Activity 4
Experiment.
Aim: To determine density of liquids using a burette.
Materials and apparatus: Burette, beaker, beam balance and kerosene.
Procedures
- Record the mass of the empty beaker m₁ using a beam balance.
- Pour the known volume of kerosene into the beaker by using bur rete, V.
- Record the mass of the beaker and kerosene m₂.
Results

Definition of the Relative Density of a Substance
Define the relative density of a substance
The Relative density
of a substance is the ratio of its density to the density of water.The
density of water has the density of approximately 1.0g/cm³ or 1000kg/m³.
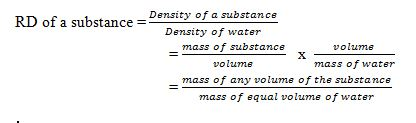
Note:Since
the density of pure water is 1g/cm³, the RD of a substance will be
represented by the same number as its density in g/cm³.RD has no units as its ratio of same quantities.
Applications of Density and Relative Density in Real Life
Interpret applications of density and relative density in real life
Application of RD in real life.
- It is the key factor which is considered during the design of various structures and equipment. Eg. ships and planes.
- Density is considered during the selection of materials.
- Density is also considered during the design of equipment used in swimming.
Force
A
force is an interaction that causes a change. In mechanics, a force is
an interaction that causes a change in velocity (an interaction that
causes acceleration).
Concept of Force
The Concept of Force
Explain the concept of force
For better understanding of this concept, let’s ask ourselves the following question:
- What causes/makes a body at rest to move?
- What causes the same body in motion to stop?
The
answers to these questions, is of course that a force is required to do
so. Here, an applied force to a body can cause the body at rest to move
or if already moving a body application of force can do the following
when applied to a body.
- Force can cause a change in the way the object moves
- Change its size or shape
- Change the direction in which an object is moving
A
force is defined as a push or pulls experienced by an object. The force
is usually described in terms of its size (magnitude) and direction.
The S.I Unit of Force
State the S.I unit of force
The SI unit of force is a Newton (N).
A Newton (1N) can be defined as the amount of force required to give a mass of one kilogram (1kg) an acceleration of 1m/s2
Measuring Instrument (Device)
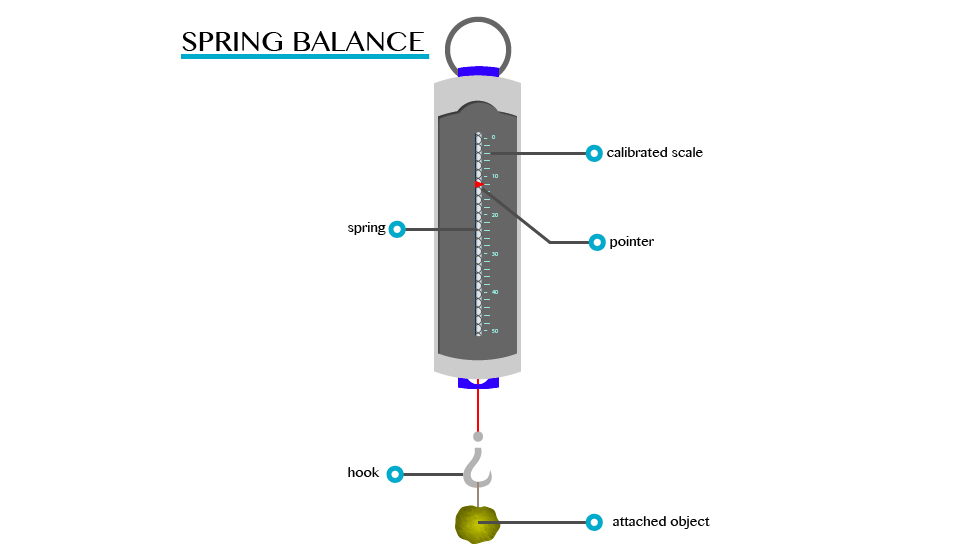
The instrument that is used to measure the amount of force that is exerted on an object is called spring balance.
A
spring balance can be used to measure small forces. It consists of a
coiled spring fixed to the other end with a hook at the other end. The
body upon which the force acts is attached to the hook. The distance
through which the spring is stretched is directly proportional to the
force applied by the balance.
A pointer is attached to the spring and the force is indicated on a calibrated scale.

Types of Force
Fundamental Types of Forces
Identify fundamental types of forces
There are two main types of forces namely:
- Fundamental forces
- Non fundamental forces
Fundamental Forces
Fundamental forces are the basic forces in nature that cannot be explained by the action of another force.
There are four types of fundamental forces. These include:
- The force of gravity
- The electromagnetic force
- The strong nuclear force
- The weak nucleus force
Force of gravity (weight)
The
force of gravity is the pull by which the earth, moon and other very
large bodies attract other objects towards themselves. It is commonly
referred to as the weight of the object that is attracted.
All
objects on the earth experience a force of gravity that is directed
downwards towards centre of the earth. The earth’s gravitational pull is
so great that it is able to hold most things firmly on the ground.
In mechanics, a freely falling body in the air moves down irrespective of its mass. This is due to force of gravity.
Example 1
A
fruit fall from a tree because the earth exerts gravitational force on
it. The force of gravity on the earth is always equal to the weight of
the object (body).
Force of gravity, W = mass (m) x gravitational acceleration (g).
W= mg
Where acceleration due to gravity on the earth, g = 9.8m/s2
Conclusion, we can say that the force of gravity has the following properties.
- It pulls (attracts) objects towards the centre of the earth.
- It is directly proportional to the mass of the object. This means that the greater the mass the greater the pull of gravity.
- It is strong when the mass is closer to the centre of the earth.
NB: It should be noted that the weight of an object is directly related to its Mass.
Weight, W = mass x g = mg
The g above has two meanings:
- It is the gravitation’s field strength (10N/Kg)
- It is the acceleration of free fall (10m/s2
Electromagnetic force
This force includes both electric and magnetic force. It is relatively strong.
Example 2
Examples where electromagnetic forces are involved include:
- In the formation of molecules of a substance. Atoms attract each other to form molecules. This is due to electromagnetic force.
- In two parallel wires carrying current. If places near each other, the electromagnetic force acts on the wires.
Strong nuclear force
This
is the force, which holds of the constitutions (sub atomic particles)
of the atomic nucleus. It acts within the nucleus of the atom.
Weak nuclear force
This is the force which appears only in certain nuclear processes.
The Properties of Each Type of Fundamental Forces
Describe the properties of each type of the fundamental forces
Properties of gravitational forces
The following are the properties of gravitational force
- It always attracts objects.
- It is the weakest force among the four basic forces.
- It is a central force (gravitational force between two objects acts along the line joining the centres of the objects.
- It operates over very long distance.
Properties of electromagnetic force include
- It may be attractive or repulsive is nature
- It is a central force
- It is stronger than gravitational force
- It is also a long-range force (operates over a very long distance)
Properties of a strong nuclear force:
- It is basically an attractive force
- It is a non-central force (does not act at the centre)
- It is stronger than gravitational force
- It is a short-range force that is it operates only up to distance of the order of 10-14m
Properties of the weak nuclear force
- It is much stronger than the gravitational force but weaker than the strong nuclear force and electromagnetic force
- It acts on small ranges of up to 10-17m
Activity 1
CLASS ACTIVITY
- Define the term force and give its SI unit.
- Mention four fundamental types of force that you know.
- Define the following terms: Force of gravity; Strong nuclear force; Electromagnetic force.
- A body has a mass of 40kg. Find its weight.
Effects of Force
The Effects of Forces
Identify effects of forces
A force acting on an object may cause the object to change shape, to start moving, to stop moving, to accelerate or decelerate.
When
two objects interact with each other they exert a force on each other,
the forces are equal in size but opposite in direction.
Resultant force
The
forces acting on an object can be replaced with a single force that
causes the object to behave in the same way as all the separate forces
acting together did, this one overall force is called theresultant force.

If the resultant force acting on an object is ZERO then:
- The object will remain stationary if it was stationary when the resultant force became zero.
- Move at a constant (steady) speed in a straight line if it was moving when the resultant force became zero.
If the resultant force acting on an object is NOT ZERO then:
- The object will accelerate or decelerate (speed up or slow down).
The Effects of Forces on Materials
Justify the effects of forces on materials
Example 3
A
rectangular bar having a cross-sectional area of 80 mm2has a tensile
force of 20 kN appliedto it. Determine the stress in the bar.
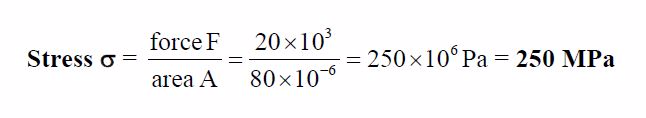
Example 4
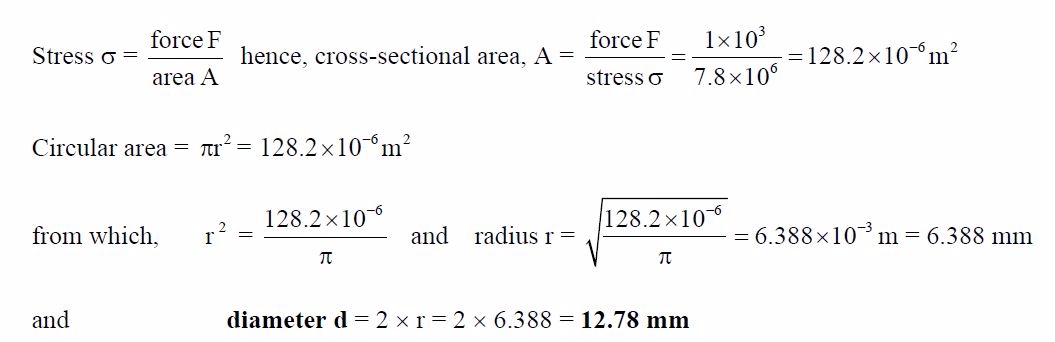
A
circular section cable has a tensile force of 1 kN applied to it and
the force produces a stress of7.8 MPa in the cable. Calculate the
diameter of the cable.

Example 5
A
split pin requires a force of 400 N to shear it. The maximum shear
stress before shear occurs is120 MPa. Determine the minimum diameter of
the pin.

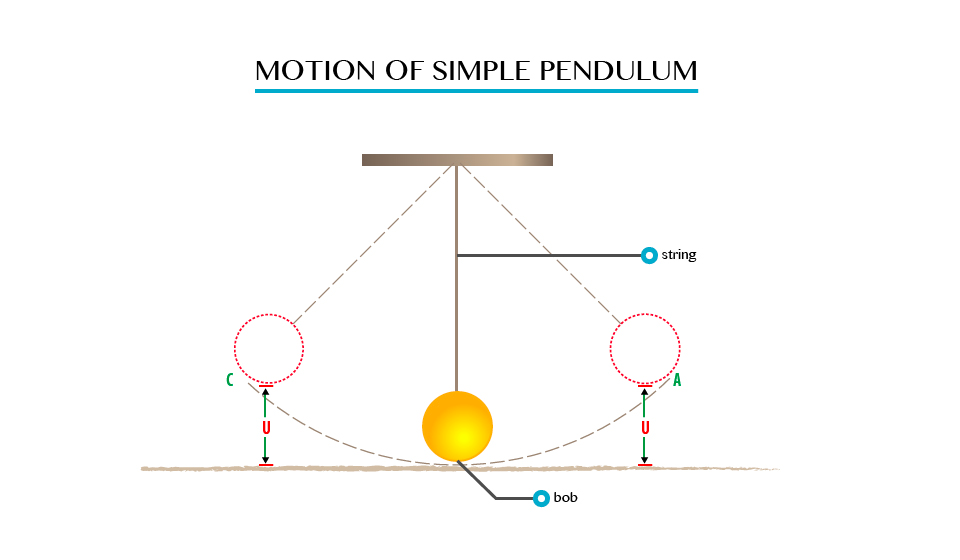
TOPIC 5: ARCHIMEDES' PRINCIPLE AND LAW OF FLOTATION
Archimedes'
principle indicates that the upward buoyant force that is exerted on a
body immersed in a fluid, whether fully or partially submerged, is equal
to the weight of the fluid that the body displaces.
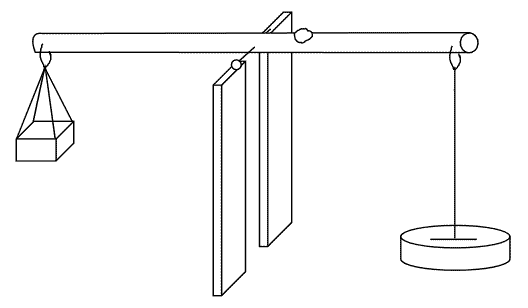
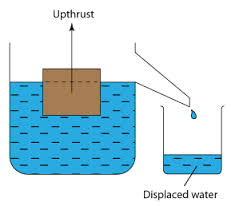
The Concept of Upthrust
Explain the concept of upthrust
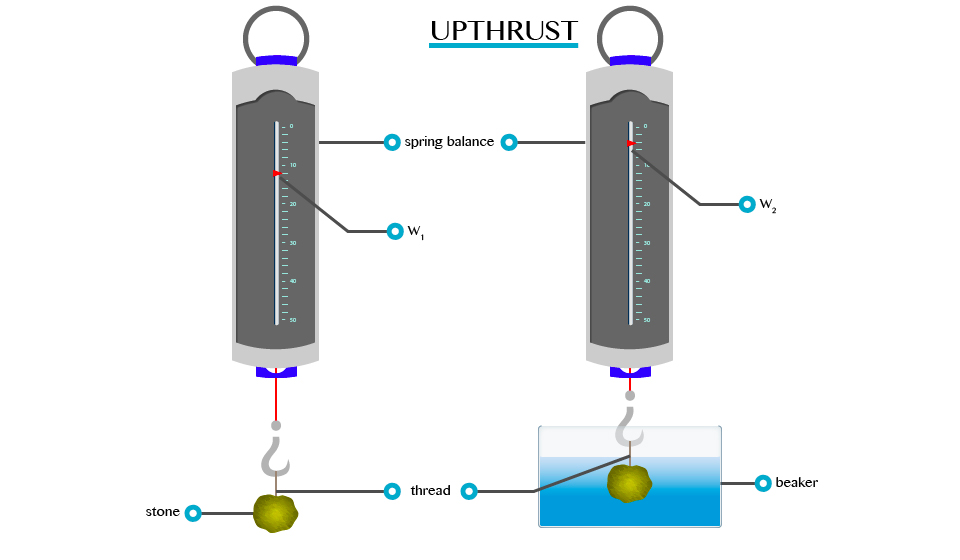
If
a heavy object is lifted while immersed in water, it may be raised more
easily than when outside the water. This is due to the reason that when
anything is placed in liquid, it receives an upward force called
upthrust. A body appears to have lost some weight when immersed in water
due to the upthrust exerted on the body by the water.
- By definition upthrust is referred as an upward force exerted by the body when it’s partially or totally immersed in water.
- Consider the experiment below to verity the concept of upthrust.
From this experiment, it will be observed that W1>W2. This is because:

When a body is partially or totally immersed in any liquid, the liquid exerts an upward force.
A
weight recorded on the spring balance of a body that is totally or
partially immersed in any liquid is called apparent weight. E.g. W2 and the force, which temporally reduces the weight of the body, are called upthrust (u).
Verification of the Archimedes' Principle
Verify the archimedes principle
This
is the principle which shows the relationship between the upthrust
acting on a body and the weight of fluid it displaces when partially or
completely immersed in the fluid. It was first discovered by a Greek
scientist called Archimedes (287 to 121 BC).
- The principle states that, ”when a body is partially or totally immersed in a fluid it experiences an upthrust which is equal to the weight of the fluid displaced.”
- This principle can be verified by the following experiment.
Results:Weight of a body in air = W1

Verification of the Archimedes' Principle
Weight of a body in water = W2 (apparent weight)
Uptrust = Loss of weight in water
U = W1 –W2
Example 1
Weight of a body in air = 10.0N
Weight of a body when immersed in water = 9.2N find the upthrust.
Solution:
Data given
Weight of a body in air (W1)= 10.0N
Weight of a body when in water (W2) = 9.2N
Formula
Upthrust = Loss of weight in water = W1-W2
= 10.0N – 9.2N
= 0.8N
The upthrust is 0.8
Example 2
The
weight of a body when totally immersed in liquid is 4.2N. if the weight
of the liquid displaced is 2.5N, find the weight of the body in the
air.
Solution;
Data;
Apparent weight (W2) = 4.2N
Weight of liquid displaced (u) = 2.5N
Weight of body in air=?
Formular;
U = W1 –W2
W1= 4.2 +2.5 = 6.7N
Weight of body in air is 6.7N
The Archimedes' Principle in Determining Relative Density
Apply the archimedes principle to determine relative density
Relative
density (R.D) of a substance can be defined as a ratio of the mass of a
certain volume of the substance to the mass of an equal volume of
water.
Relative density = Mass or weight of given volume of a substance overMass or weight of an equal volume of water.
R.D = weight of a substance in air over Weight of displaced water.
R.D = weight of a substance in air
From
Archimedes’ principle the weight of an equal volume of water is equal
to the weight of water displaced by the object, which is equal to the
upthrust loss in weight. By weighing an object in air and then in water,
the relative density can be determined.
Weight of a body in air = W1
Weight of a body in water = W2
Apparent loss in weight = W1-W2
Relative density = W1/W1 - W2
Example 3
A piece of glass weigh 1.2N in air and 0.7N when completely immersed water. Calculate its:
- Relative density
- Density of a glass
Given that density of water = 1000kg/cm3
And acceleration due to gravity = 10N/kg
Solution
Weight of the glass in air (W1) = 1.2N
Weight of the glass in water (W2) = 0.7N
R.D = W1/W1 – W2
1.2N/(1.2 – 0.7)
1.2/0.5
R.D = 2.4
R.D = Density of glass/Density of water
Density of a glass = R.D x Density of water
= 2.4 x 1000kg/cm
= 2400kg/m3
NB: Relative density has no SI unit
Law of Flotation
Difference between Floating and Sinking of Objects
Distinguish floating and sinking of objects
As
we have discussed in upthrust, different objects with different density
can sink or float. The object with higher density than water density
will sink while that object with a density lower than water’s density
will float. For example, a coin sinks in water and a large ship floats
on water.
The Conditions for a Substance to Float in Fluids
Explain the conditions for a substance to float in fluids
When
an object is completely or partially immersed in fluids, there are two
forces acting on it, the weight (W) acting downwards and the upstrust
(u) acting upwards. Refer to the figure below:
Conditions

Conditions for a body to float include:
- If W>U, there is downward movement of the body which is termed as sinking.
- If W<U, there is upward movement of the body.
- If W=U, the body is equilibrium under the action of two equal and opposite force. Thus, the body floats.
Relationship between Upthrust and Weight of Floating Body
Relate upthrust and weight of floating body
The relationship can be determined by considering the following experiment.
Upthrust and Weight of Floating Body

Principle of Floatation
State the law of flotation
The
above experiment shows that, the upthrust is equal to the weight of the
liquid displaced and therefore the upthrust is equal to the weight of
the floating body as the two forces are equal.
The
mass of the floating body is equal to the mass of fluid displaced; in
the above experiment is the same as the weight of the piece of wood.
This result is agreement with the principle of floatation.
The principle of floatation states “A floating body displaces a weight of the fluid which is equal to its own weight.”
The Law of Floatation in Everyday Life
Apply the law of flotation in everyday life
Floating of ship
Iron
is much denser than water and a block of iron sinks immediately in
water. Ships are made with hollowness such that their total densities
are less than that of water. Therefore, a ship displaces water equal to
its weights.
The
upthrust of the water is sufficient to support the weight of the ship.
When the ship is loaded with cargo it sinks lower in the water. The
volume of water displaced by the ship and its cargo depends upon whether
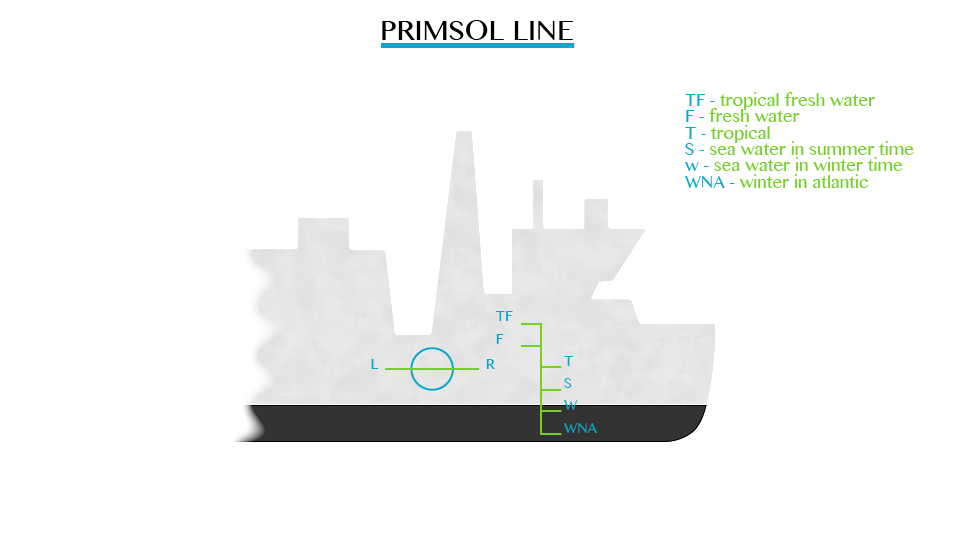
it is floating in fresh water or in seawater. It floats lower in fresh
water (R.D= 1.0) than in seawater (R.D=1.025) the mass of fresh water
displaced.
For
example, if a ship weighs 20,000 tons, then it must displace 20,000
tones of water to float. If 2,000 tones of cargo is added, the ship
lowers in water until an extra 1,000 tons of water have been displaced
Plimsoll line;This
line indicates the safe limit of loading. Many plimsoll lines may be
marked on a ship to show minimum heights above different types of water
in different seasons.

Balloons
Figure
below shows the type of balloons used to carry instruments to a high
altitude for recording meteorological measurements when filled with gas.
E.g. helium, it displaces a volume of air equal to its volume.
Air
has greater density compared to the density of a gas in the balloon.
Therefore, the weight of air displaced is greater than that of balloon.
The balloon drifts up by a force, which is equal to the difference
between the upthrust and the total weight of the balloon (W).
Submarine
The
buoyancy of a submarine depends on the quantity of water in its ballast
tanks. When it is required to drive, water is admitted to special
tanks. When the water is ejected from the tanks by means of compressed
air, the submarine raises to the surface and floats just like any other
ship.
The mode of Action of a Hydrometer
Describe the mode of action of a Hydrometer
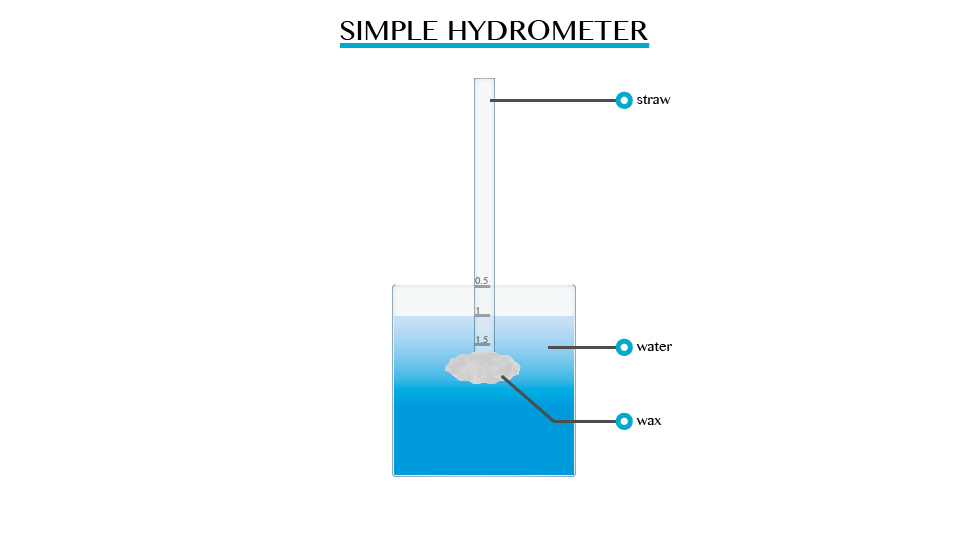
A
hydrometer is an instrument used for measuring the densities of liquids
such as milk, alcohol and acids. The greater the density of the liquid
the shorter the stem of hydrometer immersed. Hydrometer sinks lower in
kerosene and floats higher in water.
Construction of a Simple Hydrometer
Construct a simple Hydrometer
Single hydrometer can be made using pieces of straws or test tubes weighed down with wax.

Hydrometer in Determining the Relative Density of Different Liquids
Use Hydrometer to determine the relative density of different liquids
The relative density of liquid is measured directly by using a suitable hydrometer, it contains:
- A heavy sinker, containing mercury or lead shots that keep the hydrometer upright when it floats.
- An air bulb to increase the volume of displaced liquid, and overcomes the weight of the sinker.
- The stem is thin so that small changes in density give large differences in readings.
- The hydrometer is made up of glass so it does not soak up liquids.
Structure of Matter
State
of matter is defined in terms of the phase transitions which indicate
the change in structure and properties. Solids, liquids and gases all
are made up of microscopic particles. The behavior of all these
particles also varies in three phases.
The Concept of Matter
Explain the concept of matter
Matter is anything, such as a solid, liquid or gas, that has weight (mass) and occupies space. For anything to occupy space, it must have volume.
The Particular Nature of Matter
Justify the particulate nature of matter
Matter
is made up of tiny particles. The particles are atom or molecules,
examples of substances, which are made up of atoms, are: gold, copper,
Argon and silver; and those made up of molecules includes oxygen, water
and ammonia.
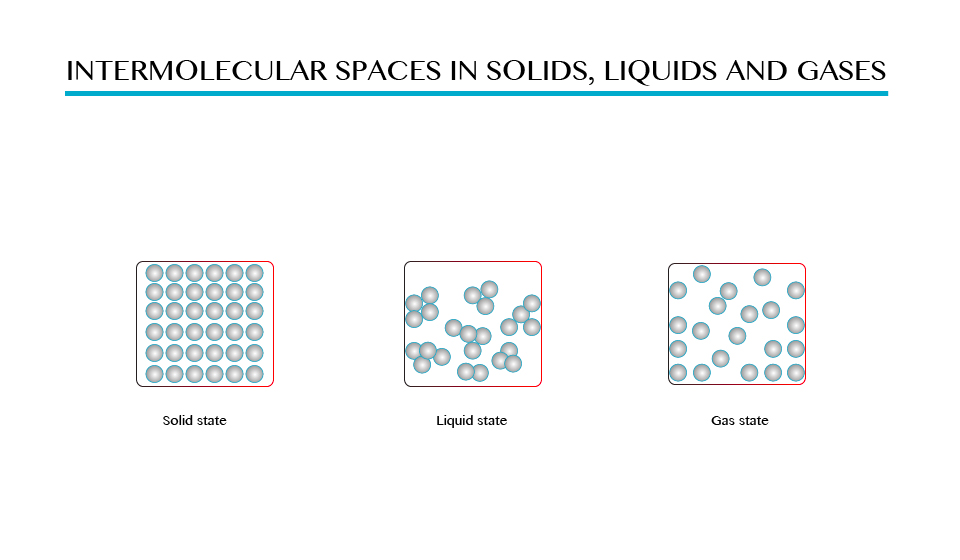
- In solid, storm’s attractive forces hold molecules together so that they are not free to move but they can only vibrate about their mean positions.
- In liquids there are weak forces of attraction between molecules therefore the molecules are free to move randomly. The distances between molecules in liquids are therefore are larger than in solids.
In
case of gases the molecules experience very weak forces of attraction
and hence they are free to move randomly filling the whole space of the
containing vessel. The distances between molecules in gases are
comparatively greater than those in solids and liquids as shown in the
figure above.

Demonstration to show the intermolecular space in solids, liquid and gases.
The Kinetic Theory of Matter
Explain the kinetic theory of matter
Generally,
when solid particles are placed in the source of lead the particles
tends to move from hot area to cold areas. These particles move because
it gains energy that called it Kinetic energy.
Kinetic
theory of matter sometimes attempts to explain how properties of gases
like pressure, temperature and volume remain in constant motion.
There are three main parts of the Kinetic theory of matter. This includes:
- Matter is made up of tiny invisible part.
- Matter comes in different sizes.
- There is a point that the smallest particles of matter can be the fastest.
Therefore
kinetic theory of matter states, “All matter is composed of small
particles”Or “Particles of matter are in steady motion and that all
impacts between the units of matter are completely elastic”
Three States of Matter
Classify three states of matter
There are three states of matter, namely:
- Solid state
- Liquid state
- Gaseous state
Solid
state is the state of matter, which include solid materials, in which
the intermolecular force between molecules are greatest and distance
between molecules is small. Examples of solid state are wood, iron, etc.
Liquid
sate is the one of the state of matter in which the intermolecular
forces are low compared to solid state, there is greater distance
between one molecule and another. See on figure 1.0 (b) examples water,
soda, kerosene, and petroleum.
Gaseous
state is the state of matter in which there is no intermolecular forces
between molecules hence molecules are free to move from one place to
another examples of gases are hydrogen, oxygen, carbon dioxide gas.
Difference between solid state, liquid state and gaseous state of matter
| Solid state | Liquid state | Gaseous state. |
| It concerns with solid matter | It concerns with liquids/ fluids matter | It concerns with gases |
| Have high intermolecular | Low intermolecular force | No intermolecular force |
| No distance between molecules | There is little distance between molecules | Molecules are far from each other |
| Good examples are iron materials, woods etc. | Good examples are water, soda, kerosene and petrol | Good examples are oxygen and hydrogen |
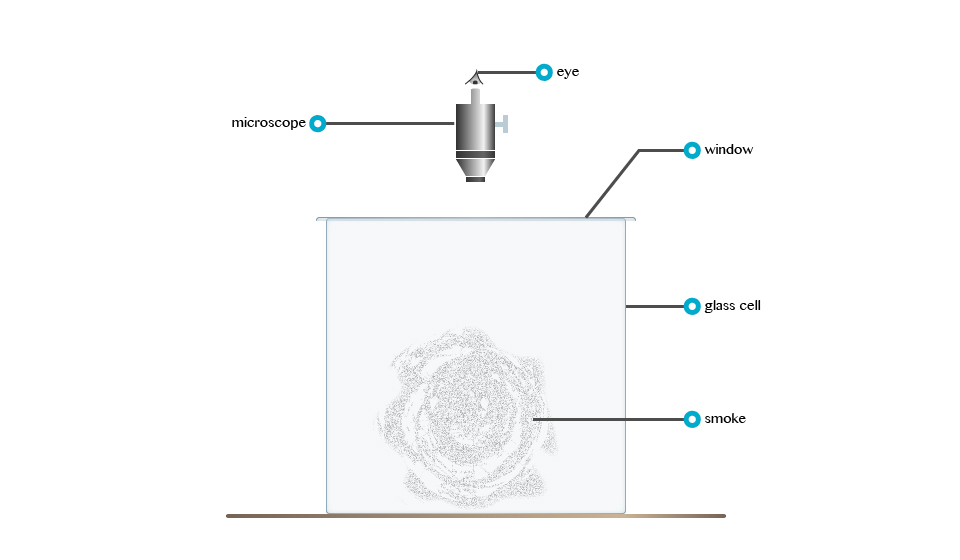
Rownian Movement
According to Robert Brown: Brownian
movement refers to the irregular motion of tiny particles suspended in
fluid (liquid organs). Consider the demonstrationbelow
Robert
Brown, an English Botanist, powered some pollen grain in water and
observed that particles floating in the water were darting about.

The irregular motion of tiny particles suspended in a fluid (fluid or gas) is called Brownian movement.
The tiny particles dart about because liquid molecules that are in state of motion bombard them.
Elasticity
The Concept of Elasticity
Explain the concept of elasticity
When
a force is applied to a body the dimension of the body is usually
altered. If an iron wire is stretched by small force applied to it
longitudinally, the wire returns to its original shape and size when the
force is removed.
Elasticity
can be defined as the property of the iron wire by which it recovers
its original shape and size on removal of the stretching force.
The Relationship between Tension and Extension of a Loaded Elastic Material
Justify the relationship between tension and extension of a loaded elastic material
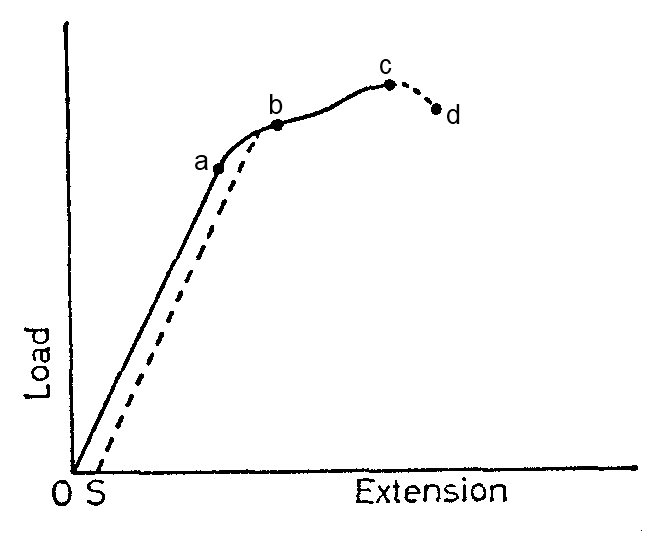
Consider the graph below:
Point A is called the elastic limit. The straight region OA of the graph has a slope K given by the ratio.

K= Tension/Extension
The ratio is called the force constant or coefficient of stiffness of the wire and it is expressed in newton per metre (N/M)
The Application of Elasticity in Real Life
Identify the applications of elasticity in real life
In
everyday life we often actually do the activities that are concerned
with the application of physics. Here are some of the application of
physics in everyday life especially in the application of Elasticity:
- Spring mattress. When you sit or sleep on a spring mattress, futon style push your weight. Pressured by the compressed spring mattress. Due to the nature of its elasticity, stretch a spring mattress again. Spring will be stretched and compressed, and so on.
- Spring that is used as shock absorbers on motorcycles. Springs used in the suspension systems of motor vehicles. The purpose of this is to dampen spring a surprise when a motorcycle driven through an uneven road surface.
- Another simple example and that you may often come across is the catapult. When it was about to shoot birds with catapults for example, rubber slingshots first stretch (given the gravity). Due to the nature of its elasticity, long rubber slingshots will return to normal after a tensile force is removed.
Adhesion and Cohesion
The Concept of Adhesion and Cohesion
Explain the concept adhesion and cohesion
Matter is made up of molecules. That exerts force of attraction. This force of attraction may be either Cohesion or Adhesion.
- Cohesion is the force of attraction between the molecules of the same substance, example water to water molecules.
- Adhesion is the force of attraction between the molecule of different substances example water to glass molecules.
Water molecules can experience the force of cohesion among themselves, where water molecules and glass molecules will experience force of adhesion.
Definite shapes of a solid are due to strong cohesion force among its molecules.
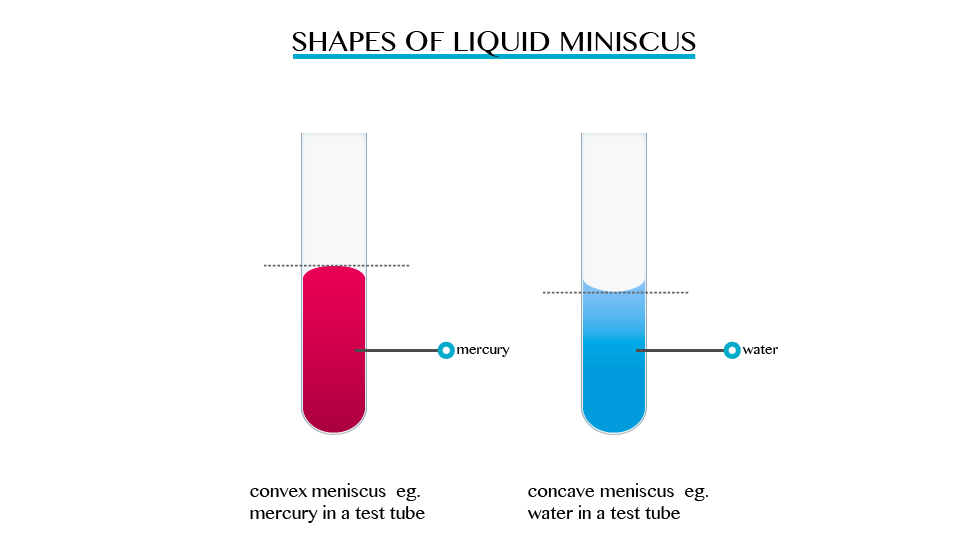
Shapes and meniscus of a liquid
When
we carried out activities involving determination of volume in a liquid
ring and measuring cylinder. The description indicated that the surface
of the liquid was carved, forming a meniscus, and that the volume must
be read at the bottom or top of the meniscus, depending on the liquid
used. For mercury, the top of the meniscus is read.
The
formation of a meniscus in a liquid is due to forces of adhesion
between the liquid and the walls of the container. The adhesion of the
liquid such as water to the wall of a vessel causes an upward force on
the liquid at the edge.

The
opposite takes place in mercury, the meniscus of water curves upwards
forming a concave shape. When a drop of each liquid, mercury and water
are placed on a glass sheet, water spreads further unlike mercury,
because of mercury’s high cohesion force among its particle.
Why water wets the glass?
Why methanol does not wet the glass?
Applications of Adhesion and Cohesion in Daily Life
Identify the applications of adhesion and cohesion in daily life
Application include the following
- To stick two different objects together. Here we use the adhesive effects of tape or glue.
- Adhesion can also be used to remove harmful materials such as bacteria from drinking water. Adhesive forces are the source attraction substance.
- Cohesion assists in transport of water in plants and animals by allowing one molecule to pull others along with it.
- The bodies of plants and animals also use the cohesion of tissue to repair damage.
- Ink sticks on paper because of adhesive force between the paper and ink.
Surface Tension
The Concept of Surface Tension
Explain the concept of surface tension
While
you may not be able to walk on water, water stride does. This is due to
the property of liquid, which is known as surface tension.
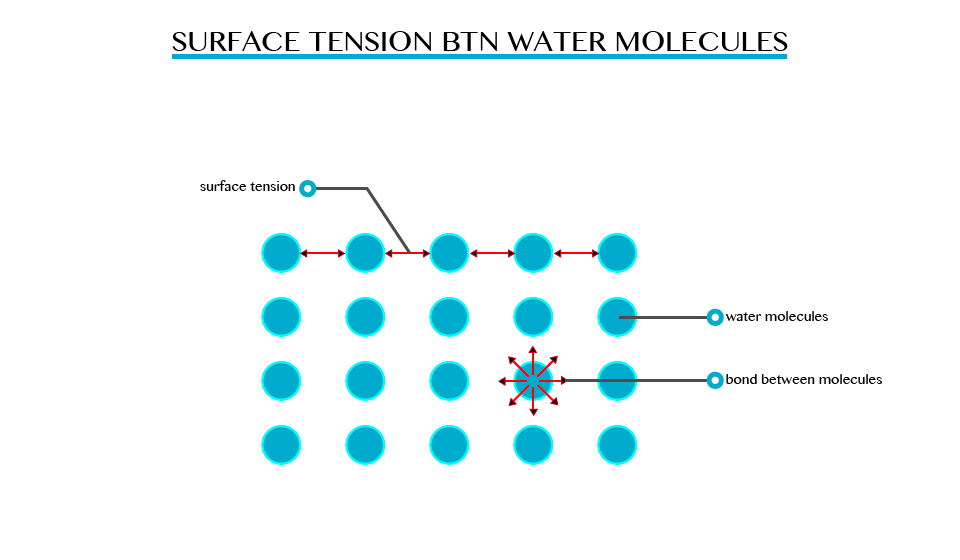
Surface
tension is the ability of the molecules on the surface of a liquid to
attract and stick to each other allowing them to resist an external
force. Surface tension enables insects such as water strides and
mosquitoes to walk on water. It allows small objects even metallic ones
such as needles and razor blades to float on the surface of water.
Surface
tension is a resultant attractive force between molecules in a liquid.
The molecules below the surface liquid have forces of attraction between
neighbouring particles. However molecules at the surface have no
neighbouring molecules above them. This makes them have stronger
attractive force than their nearest neighbours on the surface.
However,
when some detergent is added to water, the same objects sink to the
bottom of the trough. This means that the detergent interfered with the
surface of the liquid so decreasing the tension of the water surface.

Detergents are example of surfactants. A surfactant is a substance that reduces the surface tension of a liquid.
Note: the term surfactant is an aerogun for surface-active agent.
Surface tension is affected by the following
- Nature of the liquid
- Contamination/impurities
- Temperature
Application at surface tension:
- In extraction of impurities dating laboratory process.
- Surfactants are also used to make emulsion of liquid like oil and water.
- In cleaning action of soap.
Applications of Surface Tension in Daily Life
Identify the applications of surface tension in daily life
Application at surface tension
- In extraction of impurities dating laboratory process
- Surfactants are also used to make emulsion of liquid like oil and water.
- In cleaning action of soap
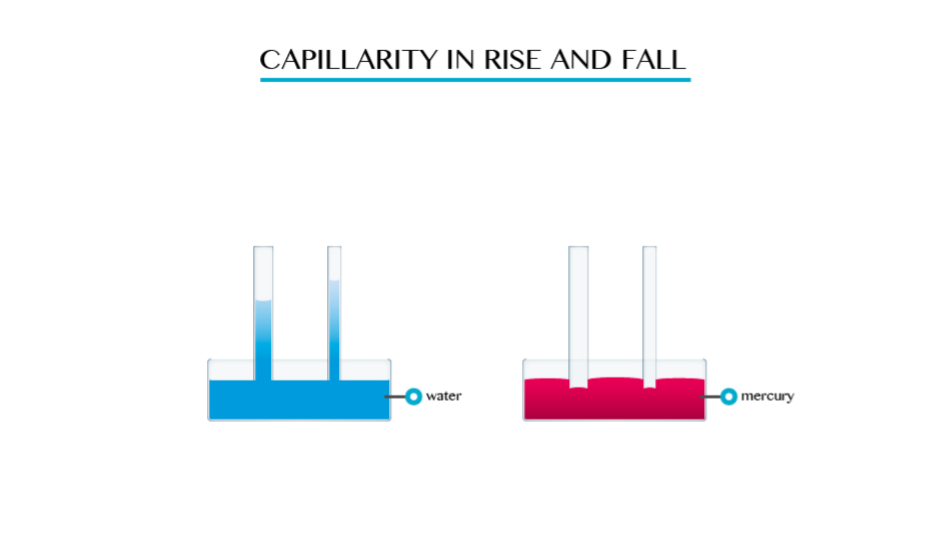
Capillarity
The Concept of Capillarity
Explain the concept of capillarity
This
is the tendency of a liquid to rise in narrow tubes or to be drawn into
small openings such as those between the fibres of a towel. Capillarity
can pull a column of liquid upward until the weight of liquid becomes
greater than the surface tension.
In a tube, capillarity depends on the tube’s diameter but weight of water column depends on other factors besides it.
The
smaller the radius of the tube the higher the liquid will rise in it.
This implies that capillarity height is immensely proportional to the
diameter of the tube.
By definition
Capillarity
is defined as the tendency of liquid to rise in narrow tubes or to be
drawn into small openings such as those between the fibres of a towel.
Capillarity action is the ability of a liquid to raise or fall in a narrow tube.
Note:

- Capillarity depends on the type of liquid. For example if you dip capillarity tube in water the water rises in the tube and above the level of the water in the vessel.
- If the tube is dipped in mercury, the liquid does not rise in the tube. It suffers capillarity depression.
Applications of Capillarity in Daily Life
Identify the applications of capillarity in daily life
The application includes:
- Capillarity is essential to plants and animals.
- In plants, it facilitates the transport of water and nutrients from the roots to the leaves where photosynthesis produces the plants food. In animals it assists in the circulation of blood.
- Capillarity promotes the movement of ground water.
- It is the principles on which paper and fabric towels work to absorb water.
- Cotton clothing in hot climates uses capillarity action to draw perspiration away from the body.
- In an oil or kerosene lamp capillarity draws the fuel up into the wicker where it can be burnt.
- A writing Rubin splits in the middle so that a fine capillary is formed.
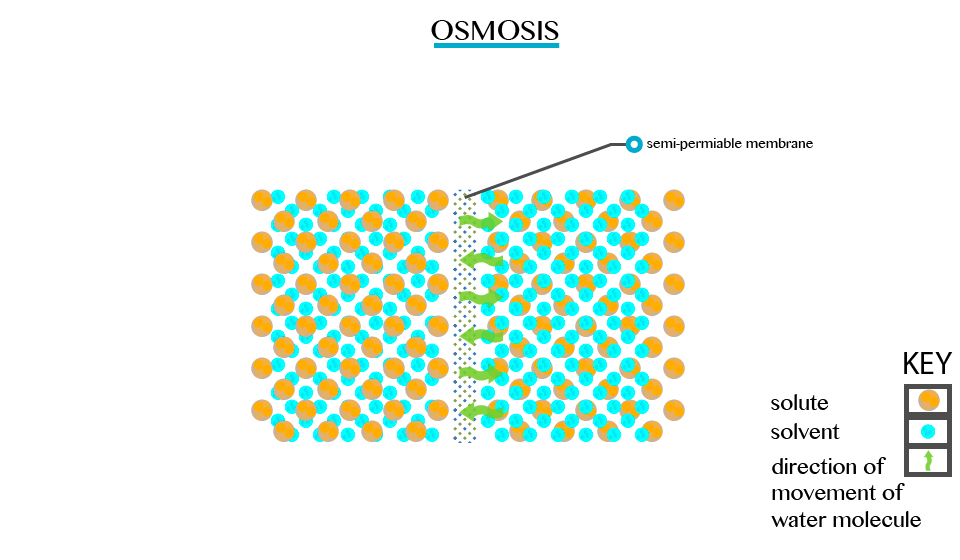
Osmosis
The Concept of Osmosis
Explain the concept of osmosis
Defined as the movement of a solvent from a region of low concentration through semi permeable membrane.
Particles
will diffuse through the membrane in an attempt to equalize the
concentration on either side. E.g. two solutions of different
concentration separated by a semi permeable membrane. The membrane is
permeable to the smaller solvent molecules but not to the larger solute
molecules. Osmosis stops when the concentration becomes the same on
either side of the membrane.
Osmosis stops when the concentration becomes the same on either side of the membrane.

Applications of Osmosis in Daily Life
Identify the applications of osmosis in daily life
Applications of osmosis in daily life:
- Control the movement of water and nutrients in and out of the cell.
- Filtration processes.
- Removal of harmful ingredients from dinking water.
- Removing salt from seawater so as to make it suitable for drinking and for other domestic uses.
TOPIC 7: PRESSURE
Concept of Pressure
The Concept of Pressure
Explain the concept of pressure
Pressure
is defined as the force per unit area. OR Pressure is the force acting
normally (perpendicularly) per unit surface area.
It is calculated by the formula:
Pressure = Force (f)/Area (A)
P = F/A
Where
- P – Pressure
- F – Force
- A – Area
The S.I Unit of Pressure
State the S.I unit of pressure
The SI unit of Pressure is Newton per square metre (N/M2). This unit is usually referred to as the Pascal (Pa).
1Pa = 1 N/M2
The other units of pressure are atmosphere, torr bar and mmHg.
- 1 atmosphere = 780mmHg
- 1 atmosphere = 1 105 N/M2 = 1bar (used by meteorologists)
Note: for a given amount of force, the smaller the area of application the greater the pressure exerted.
When
a man lifts a bucket of water by its handle that is made with a thin
metal, he would experience some discomfort but if the bucket was made
with a thicker handle the discomfort will be much less if any.
This is because the area over which the force is applied is larger.
Pressure due to Solids
Dependence of Pressure on Surface of Contact
Explain dependence of pressure on surface of contact
The
pressure in solid depends on the surface area of contact. A force (F)
applied onto a small area exerts a higher pressure as compared to when
it is applied onto a large surface.
Pressure in solid = Force applied/Area of contact.
Example 1
A
block of wood that weighs 30N and measures 5m by 10m by 4m. If it was
placed on a table with the largest possible area (5mx10m) in contact
with table, exerts less pressure than it would when placed with its
smallest possible area (5mx4m) in contact with table.
Solution
Data:
Force = 30N
Largest base area = ?
AL = 10mx5m = 50m2
P = F/A = 30N = 0.6N/30m2
Pressure = 0.6N/m2
Force (F) = 30N.
Small base are = ?
As = 5mx4m
As = 20m2
P= F/A = 30N/20M2 = 1.5 N/M2
Pressure = 1.5 N/M2
Example 2
A tip of needle has a cross- sectional area of 1x106m2.
If doctor applies a force of 2N to a syringe that is connected to the
needle, what is pressure is exerted at the tip of the needle?
Soln:
Data:
Area (A) = 1x106m2
Force (F) = 20N
Pressure =?
P = F/A
P = 20 = 2.0x107 N/M2
The pressure extended by the needle lip is 2.0x107N/m2
Example 3
A
rectangle metal block with sides 105m by 1.0m by 1.2m rests on a
horizontal surface. If the density of the metal is 7000kg/m3. Calculate
the maximum and minimum pressure that the block can exerts on the
surface.
(Take the weight of 1kg mass to be 10N)
Solution:
Data:
Dimension = 1.2m,1.5m,1.0m.
Density = 7000kg/m3
Maximum pressure (Pmx) = ?
Minimum pressure (Pmn) = ?
Volume = 1.2x1.5x1=18m3
Density = mass/ Volume
7000kg/m3 = mass/18
Mass = 7000x18 = 126000Kg
1kg = 10N
126000Kg = ?
F = 1260000 = 1.26 x 106
Force of metal = 1.26 x 106
A1 = 1.2 x 1.5= 18m2
A2= 1.2m2= 1.2mx1m=1.2m2
A3= 1.5 x 1m= 1.5m2
Amx = 18m2
Amin = 102m2
Pmn = F/Amx =1.26 x 106N/18M2 = 1.26X106/1.8 X101
Maximum pressure = 1.05x106N/m2/maximum pressure= 0.7x105N/m2
The Applications of Pressure due to Solids
Identify the applications of pressure due to solids
It
is used to make different objects like screw, nails, pins, spears and
arrows. This item is given sharp points to increase their penetrating
power.
It helps some of living organisms for self-defence. A fish uses its sharp fins to protect itself.
When one walks on shoes with sharp pointed heels they exert greater pressure on the ground than when wearing flat shoes.
It
helps in construction of railways. During the constructions of
railways, wide wooden or concrete sleepers are placed below the railways
tracks. This provides a larger surface area over which the weight of
the train acts. This is a safety measure to train tracks.
Buildings are constructed with wide foundations to ensure that the weight of the building acts over the layer area.
Pressure in Liquids
A
liquid will exert pressure on an immersed object as well as on the
walls of the container holding it. Note that the pressure exerted by
liquids is due to the weight of the liquid. Also increase in water level
in liquid cause increase in pressure.
The Characteristics of Pressure in Liquids
Describe the characteristics of pressure in liquids
Note: Pressure in liquids =Force/Area
But force= hApg/A
=Hsg
Hence pressure in liquids is given as hsg.

H= Height of the liquid column
A= Area of the base
The pressure at any point in a liquid at rest then depends
- Depth
- Density of liquid
Note: It does not defend on the area
Pressure in liquids is characterized using the following parameters
- Pressure in a liquid increase with depth
- Pressure in a liquid acts equally in all directors
- Pressure in a liquid increases with increase in density of the liquid.
Mercury exerts more pressure than an equal volume of water. This because mercury is denser than water.
The Variation of Pressure with Depth in Liquids
Examine the variation of pressure with depth in liquids
Demonstration of water spurting from holes at different heights.

As
pressure at point A is low due to small height of water above it but at
point B the height increase and the distance of water increase while at
point C the height is greatest therefore the level of water distance
will be large distance.
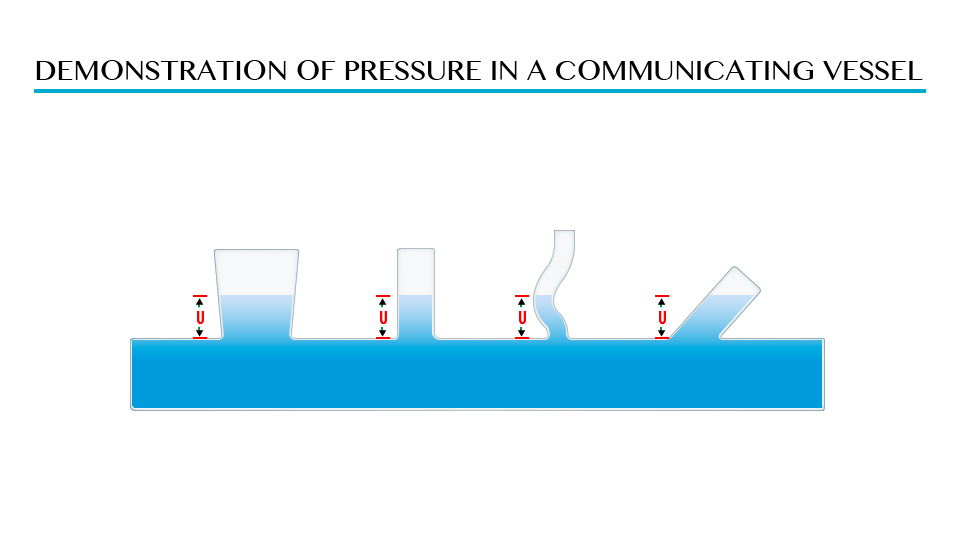
Demonstration of pressure in a communicating vessel

The
shape of vessel found in point A, B, C and D is different but the
pressure is the same due to the equal height (L) of the liquid above the
points.
Problems Involving Pressure in Liquids
Solve problems involving Pressure in Liquids
Example 4
A cube of side 2cm is completely submerged in water so that the bottom of the cube is at a depth of 10cm. use g=10m/s2 and s=1000kg/m3
- What is different between the pressure on the bottom of the cable and the pressure on its tap?
- Determine the difference in the force on the top and bottom.
- What is the weight of the water displaced by the cube?
Solution;
Data.
Depth = 10cm = 0.1m
s = 1000kg/m3
g = 10m/s
h= 8cm= 0.08
formular
p= sgh
p=1000kg/m3x10m/s2x0.1m=1000N/m2
At the h= 0.08m p= 1000x10x0.08= 800N/m2
Change in pressure = 1000N/m =200N/m2
So that different in pressure between the bottom the top is 200N/m2
Pressure = Force/Area = P= F/A
Area =L2= (10.02)2= 0.0004m2
Distance in water 200N/m2x0.0004m2
This is the upthrust acting on the cube
Water displaced by the cube in weight= volume of the cube
Volume = l3 (2cm) 3 = 8cm3
For water, 1cm3= 1g 50.8cm3 = 8g
Weight of displaced water
mass of displaced water/100=8g = 0.08N
Example 5
Calculate the pressure at the bottom of tank of water 15m deep due to the water above it is (s=1000kg/m3).
Solution:
Data:
Height =15m
S=1000kg/m3
g= 10m/s2
Formula
Pressure in liquid sgh
= 1000x10x15=150000N/M2
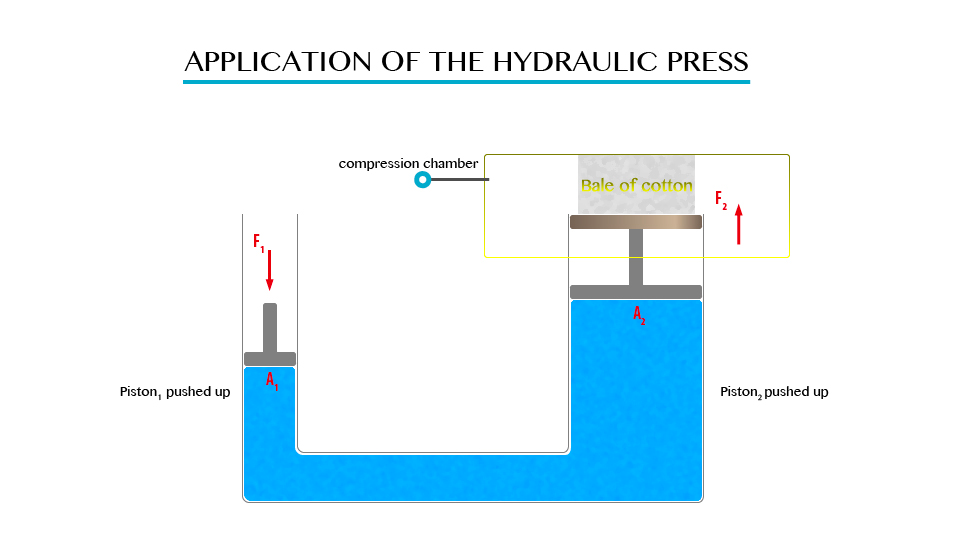
The Principle of a Hydraulic Pressure
Explain the principle of a hydraulic pressure
It
state that “Any external pressure applied to the surface of an enclosed
liquid will be transmitted equally throughout liquid.”
Illustration of Pascal’s Principle.

Note: in all side of vessel will experience equal pressure.
The
hydraulic press works on Pascal’s principle, when using this principle a
small force converted into a large force and vice versa.
From Pascal’s Principle
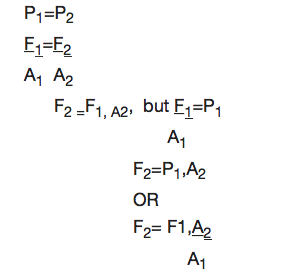
Note: The distance moved (d) is inversely proportional to the cross sectional area.
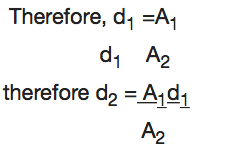
Example 6
The pistons of a hydraulic press have their areas given as 3.0x10-4m2 and 2x102m2 respectively. If the smaller piston is pushed down with a force of 12N, what is the force required to push the larger piston?
Soln:
Data:
F1 = 120N, A2= 2X102m2
A2 = 3X104m2
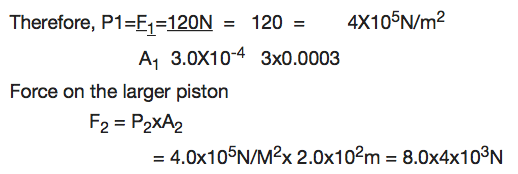
Application of Hydraulic Press
Measure pressure of a liquid
Hydraulic press is used in industries to express bulk items.

Hydraulic brake system
When
pressure is applied to the brake pedal, it pushes the piston in the
master cylinder forward creating a pressure in the brake fluid. This
pressure is transferred to the slave cylinders where it is multiplied
and pushes the brake shoes against the brake dram that is attached to
the wheel of the vehicle.

It is used in industries in the forming of metals.
Since the hydraulic press act like a lift, it is used for lifting heavy loads.
Measuring the pressure of liquids using a manometer. Manometer is the device for measuring pressure (commonly gas).
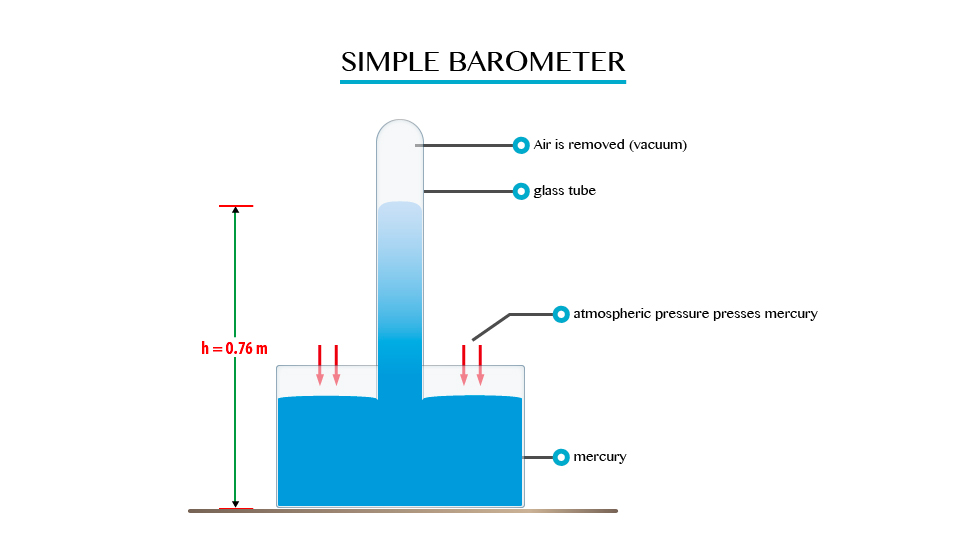
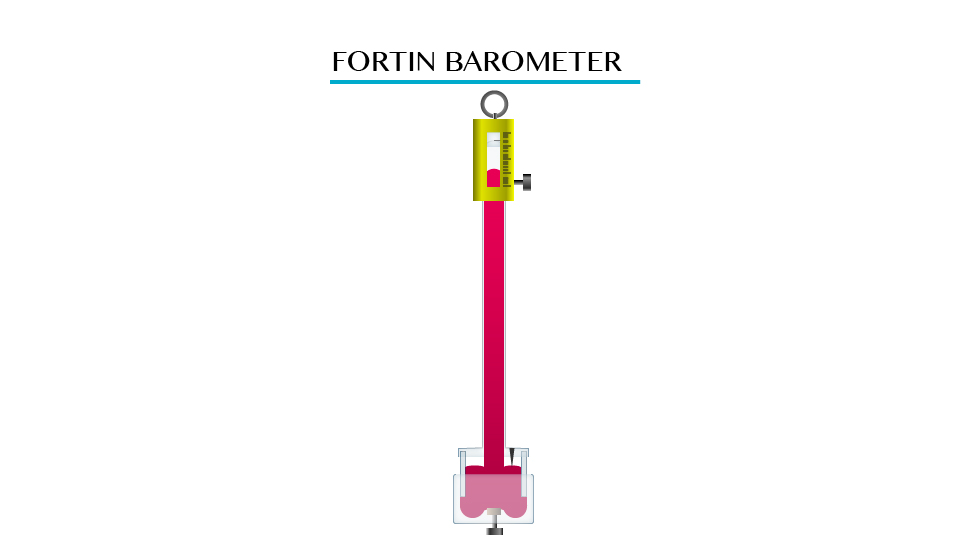
Atmospheric Pressure
The Existence of Atmospheric Pressure
Describe the existence of atmospheric pressure
Atmospheric
pressure is as a result of the weight of this layer of gases, the act
at atmospheric pressure is surrounded the layer of air consisting of a
mixture of gases.
Note. The atmospheric pressure on the earth’s surface and objects on the earth is a approximately 1.01x105N/m2.
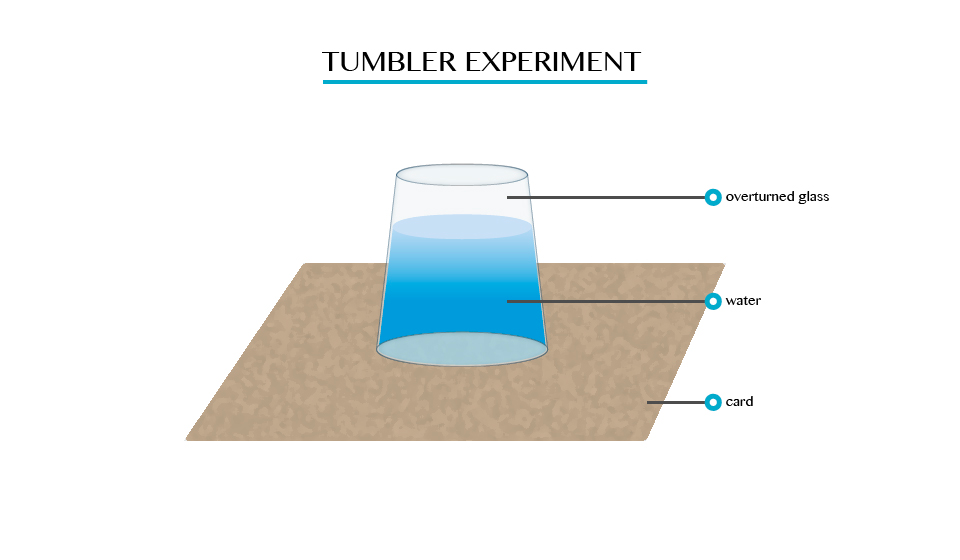
The experiment which demonstrate the existence of atmospheric pressure.
Umbler experiment

When you fill a glass timber with cord and gentle turned upside down. It will be seen that water will not poured down.
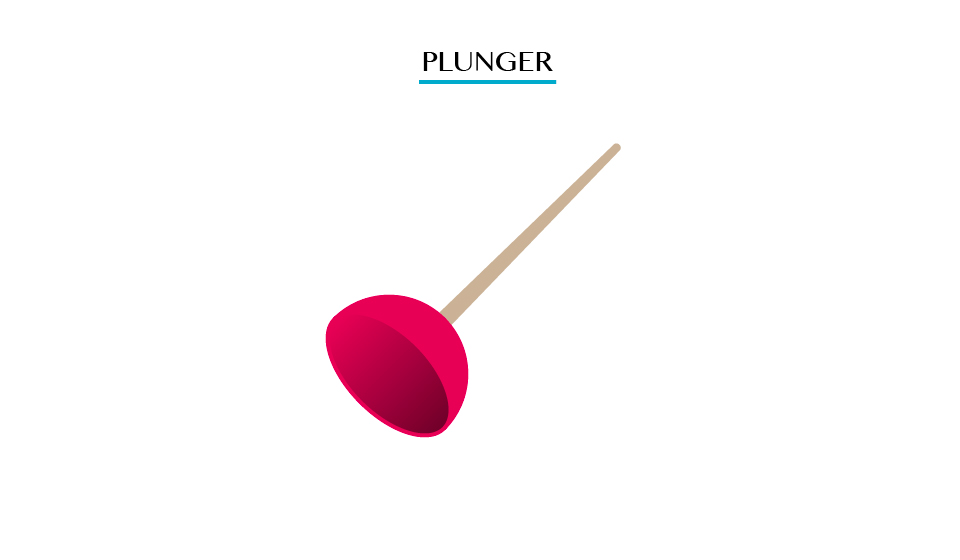
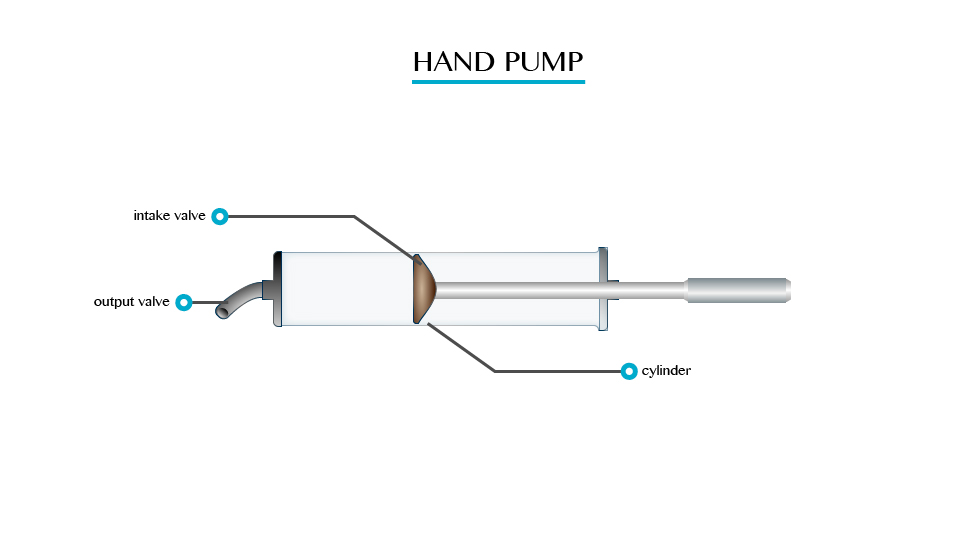
Plunger

Pulling
the plunger is not easy. This is so because all the air is squeezed out
from the table when the surrounding pressure being high the plunger
sticks.
Crushed bottle

When
putting hot water in, the bottle is cooled under cold water the steam
condenses; leaving partial vacuum inside the bottle consequently the
greater atmospheric pressure outside the bottle crushes it in wards.
Applications of Atmospheric Pressure
Identify the applications of atmospheric pressure
A siphon

A siphon is applied in areas and devices that we use everyday
- It is used in the toilet flushing cisterns (chain and ball tank). The flush is triggered by a handle that operates a simple diaphragm like piston pump that lifts enough water.
- It is used in special rain gauges called siphon rain gauge which are able to automatically drain out excess water.
- A siphon cup is a reservoir attached to a gum.
- It is used is some drainage systems to drain water to one point.
The lift pump

A
lift pump is used to raise water from underground sources. This is a
pump that is used to lift the liquid, rather than force liquid up.
A
syringe is a simple piston pump that lifts a tube. The plunger that
lifts can be pulled and pushed through inside a cylindrical tube or
barrel. This enables the syringe to take in or expel fluid through the
opening (nozzle) at the end of the tube.

Uses of syringe:
- They can be fitted with hypodermic needles and used to administer injections.
- They are used to measure liquids and gases in a laboratory.
- They are used to apply certain compounds such as a glue or lubricant.
Bicycle pump
This is a type of force pump that consists of a hollow metal cylinder and a movable piston.

Meassuring Atmospheric Pressure
Measure atmospheric pressure
Atmospheric pressure is measured by using barometer;
Types of barometer:
- The simple barometer
- Fortin barometer
- Aneroid barometer
This is a type of force pump that consists of a hollow metal cylinder and a movable piston.
A simple barometer is the most fundamental of the other types of barometer. The barometric liquid used is mercury.
A simple barometer consists of a hard glass tube closed at one end.

Fortin barometer
A
Fortin barometer is a modified simple barometer. It consists of an
inverted tube closed at its upper end with the lower open end immersed
in a reserve of mercury. The atmospheric pressure is measured in terms
of the height of the column of mercury.

Disadvantages of Fortin barometer:
- Mercury is very expensive to use and is very toxic. For these reasons, an aneroid barometer is usually preferred.
- It is not portable as it is generally big and contains liquid.
- It must be mounted in a vertical position.
Work
The Concept of Work
Explain the concept of work
If
a person pushes a wall and the wall does not move, though the person
may sweat and physically become tired, he would not have done any work.
But if the person pushes a trolley and the trolley moves it is said work
is done.
The S.I Unit of Work
State the S.I unit of work
Work is the product of force and distance moved in the direction of the force.
Thus,
Work = Force (f) * distance (d) moved in the direction of the force.
SI unit of work is Joules.
The Work Done by an Applied Force
Determine the work done by an applied force
Example 1
A sack of maize, which weighs 800N, is lifted to a height of 2m. What is work done against gravity?
Data given:
Force (f) = 800N
Height distance = 2m
Work done = ?
Solution:
Work done (w.d) = force (f) x distance (d)
w.d = 800N x 2m
= 1600 Joules
Work done (w.d) = 1600Joules
Energy
The Concept of Energy
Explain the concept of energy
Energy can be defined as capacity of doing work.
Energy has the same SI unit like that of work, and that is Joules (J)
S.I Unit of Energy
State S.I unit of energy
Energy has the same SI unit like that of work, and that is Joules (J)
Different Forms of Energy
Identify different forms of energy
There are different forms of energy such as:
- Chemical energy
- Heat energy
- Light energy
- Sound energy
- Electrical energy
- Nuclear energy
- Solar energy
Difference between Potential Energy and Kinetic Energy
Distinguish between potential energy and kinetic energy
There are two types of chemical energy, which are:
- Potential energy:It is the energy possessed by a body mass in its position or state.
- Kinetic energy: It is the energy possessed by a body due to its motion.
Consider when the body is vertically thrown upwards with an initial velocity ‘u’ from the ground.

At the ground:The height is zero and initial velocity is at maximum so as to attain maximum height.
Therefore K.E = ½ MV2 will be maximum
K.Emax = ½ mv2
Where K.E = Kinetic energy
M = Mass of the object/body
V=Velvety
P.E = Mgh
Where
P.E = Potential energy
M= Mass of the object
H = Height of the object
g = gravitation force
P.E = Mgh will be zero because P.E
= M*g*0 (body at the ground where k=0)
Neglecting
the air resistance, as the body moves upwards its velocity decreases it
also experiences gravitational force (g) pulling downwards towards the
earth’s centre.
The maximum Height Attained
The final velocity of the body will be zero (V=0)
Therefore K.E = ½ mv2
K.E = ½ m(0)2
K.E = 0
P.E = MgHmax
Note:That
the object drops from Hmax that is; it leaves with zero Kinetic Energy.
At position A in fig. 8. The conservation of mechanical energy (M.E) is
given as:
P.E + K.E = Constant
(The sum of P.E and K.E is constant throughout the motion of the object if the air resistance is neglected)
The Transformation of Energy
Explain the transformation of energy
The notion of energy is that energy is changed from one form into different forms using transducers.
Transducer is a device used to transform energy from one form to another.
For example:
- Battery converts chemical energy into electrical energy.
- A generator converts mechanical energy into electrical energy.
- A motor converts electrical energy into mechanical energy.
The Table Summarising Energy Transformation
| ORIGINAL ENERGY | TRANSDUCER | ENERGY TRANSFORMED |
| Chemical energy | Battery | Electrical energy |
| Chemical energy | Motor | Chemical energy |
| Mechanical energy | Generator | Electrical energy |
| Solar energy | Solar panel | Electrical energy |
| Chemical energy | Motor car | Mechanical energy |
| Electrical energy | Microphone | Sound energy |
| Electrical energy | Heater | Heat energy |
Activity 1
To demonstrate pressure of potential energy.
Materials and Apparatus
- A heavy stone
- A bucket full of water
- A strong inelastic rope; and
- Smooth pulley
Procedures:
- Collect the heavy stone, using a strong rope tie it to a bucket of water
- Pass the rope over smooth pulley fixed to a support.
- Hold stationary the heavy stone at height “h” above the ground
- Release the stone

Results and observations:
- When the stone released the bucket of water will start to rise.
- The stone is said to have potentials energy because of its position above the ground.
- Lifting a body of mass “m” to a height “h” above the ground requires work to be done against gravity. Work = Mgh
Example 2
A ball of mass 0.5 kg is kicked vertically upwards and rises to a height of 5m. Find the potential energy by the ball.
Data given:
Mass of the ball (Mb) = 0.5 kg
Height (h) = 5m
Gravitation force (g) 10N/kg
Potential energy (P.E) = ?
Solution:
Potential energy (P.E)= mgh
= 0.5kg x 10N/kg x 5m
= 25 NM
1NM= 1Joules
Potential energy (P.E) = 25 Joules.
Activity 2
Aim: to investigate the law of conservation of energy by a simple pendulum.
Materials and Apparatus:A pendulum bob and light inelastic string.
Producers
- Pull the bob of a simple pendulum in position A so that it is at height “h” above position B.
- Release the bob so that it swings to position C via the lowest position B and back to A.
- Consider the figure below:

Observation
When the bob is at position A, it possesses potential energy only due to the height “h” which is equal to “Mgh”.
As it swings downwards to position B, the height decreases, and as the result it loses potential energy.
- The bob has Vmax and hence K.Emax at B. The height at B is zero, thus the P.E is zero.
- As it swings towards C, the P.E increases and reaches its maximum again in position C, where the Kinetic Energy is zero. At position D, the energy of the bob is party potential and party Kinetic.
The Principle of Conservation of Energy
State the principle of conservation of Energy
The
law of conservation of energy state that “ Energy can neither be
created nor destroyed but can only be converted from one form to
another.”
This means the amount of energy is constant all the time.
Example 3
A
stone of mass 2kg is released form a height of 2m above the ground.
Find the potential energy of the stone when it is at the height of 0.5m
above the ground.
Data given;
Mass of the stone (Ms) = 2kg
Height released (h) = 2m
Gravity (g) = 10N/kg
Potential energy = (P.E) ?
Solution:
P.E at height g 2m
P.E = Mgh
= 21g x 10N/kg x 2m
P.E = 40 Joules
P.E at 2m = 40Joules
Than P.E at height of 0.5m
= 21g x 10N/kg x 0.5m
P.E at 0.5m = 10Joules
100s of P.E = 40 Joules - 10Joules
= 30 Joules
According
to conservation of energy the loss of P.E should be equal to the gain
in K.E, when the air resistance is neglected.
K.E of the stone at 0.5 above the ground = 30 Joules
Example 4
A
ball of mass 0.21kg is dropped from a height of 20m. on impact with the
ground it loses 30J of energy. Calculate the height which it reaches on
the rebound.
Data given;
Mass of ball (Mb) = 0.2kg
Height dropped (h) = 20m
Loose in energy (E) 30J
Height which reaches=?
Solution;
Consider the figure below;

At 20m above ground the initial energy of the ball = Mgh
= 0.2kg x 10N/kg x 20m
= 40 Joules.
So after the impact the ball loose 30J and the energy remaining is 40 J-10J
= 10Joules
At the top of rebound the energy of the ball = potential energy (P.E)

The height reaches (h) is 5m.
Uses of Mechanical Energy
Explain the uses of mechanical energy
The
mechanical energy can be used to produce electric power using
generators. Some uses of mechanical energy are: It enables our body to
do work, it makes work easier and faster, it is used to transport goods
and people from one place to another, many transport vehicles uses the
knowledge of mechanical energy. Examples of vehicles which uses
mechanical energy are aeroplanes and motor cars.
Power
The Concept of Power
Explain the concept of power
Power is the rate of which work is done.
- It is a measure of the rate at which energy changes.
- This means that whenever work is done energy changes into a different form.
The S.I Unit of Power
State the S.I unit of power
The SI unit of power is Jules per second J/S or watts, W.
1 Joules per second = 1 watt
When 1 Joules of work is done per second the power produced is a watt. Watt is the unit for measuring electrical power.
The Rate of Doing Work
Determine the rate of doing work
Suppose
that two cranes each lift objects having masses of 200kg to a height of
12m. Crane A lifts its object in 10sec while crane B requires 15sec to
lift its object. Assume they lift the objects at a constant velocity
they do the same amount of work.
Work done = GPE
= Mgh
= (200kg) (9.8m/s2)(12m)
= 23520J
Each did a work that was equivalent to 23520J.
What is different for the two cranes is the rate at which they did the work or their generation of power.
The power of crane A can be calculated by;

PB = 1568 watts.
Example 5
How much power is required to accelerate a 1000kg car from rest to 26.7m/s in 8sec?
Solution:
The work done on the car increases its Kinetic energy.
Work done = AKE
½ MV2 – ½ MV2
The power required is given by:
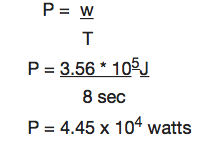
Example 6
Car engine is rated in horsepower (hp) where 1hp = 746watts. What is the required power measured in horsepower?
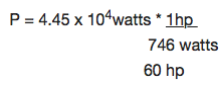
Since work causes a change in energy. DE power can be considered as the rate of change of energy.
P = DE/t
Sources of Light
The Concept of Light
Explain the concept of light
Light
can carry both energy and information. It both is the way the sun
delivers energy to the world, making life possible, and it is a very
important way that we learn about the world, through our sense of sight.
Sources of Light
Identify sources of light
The
sun is the natural source of light. On the other hand, torch, candle,
kerosene lamp, electric bulb, Florescent lamp, etc are some common
examples of artificial sources of light, bodies which give out light are
called Luminous bodies .
Difference between Luminous and Non-luminous Bodies
Distinguish luminous from non-luminous bodies
Non-luminous
bodies are bodies which do not produce light, non-luminous bodies are
seen because they reflect light from luminous bodies in such a way that
our eyes receive light from travel in a straight line. Due to
transmission of light, we are able to see.
Propagation and Transmission of Light
The Concept of Rays and Beam of Light
Explain the concept of rays and beam of light
A
straight line showing a path of light is called a ray which is the
direction of the path taken by light. Rays of light are represented in
diagrams by full straight line with arrow marking the direction in which
the light is travelling
A
beam is a stream of light rays, and it is usually represented by a
number of rays. Rays of light can converge (converging rays) or they can
diverge (diverging rays)
A ray of light

Parallel rays

Diverging rays

Converging rays

Verification that Light Rays Travel in Straight Line
Verify that light rays travels in straight line
Activity 1
Aim: To demonstrate the rectilinear propagation of light.
Materials and apparatus
- The pieces of card board with a hole in each.
- Source of light, eg. a candle.
Procedures:
- Put the pieces of cardboard with the holes in a straight line.
- Arrange them to a distance of about 50cm apart.
- Place a lighted candle at one end of cardboard A. observe the light from behind cardboard C.
- Now displace any one of the pieces of cardboard, eg. Cardboard B.

Propagation of light in straight line.
Observations
When
observing from card C, the candle can be seen. Displacing any one of
cardboards, the candle can no longer be seen as the holes are no longer
in a straight line. This happens due to the fact that light travels in a
straight line.
Transparent, Translucent and Opaque Materials
Identify transparent, translucent and opaque materials
Objects
can be grouped according to transmission of light through them, ie. The
degree passage of light as light rays falls on their surface.
Opaque bodies
These are bodies which do not allow light to pass through them, ie. Stones, wood, concrete walls and bodies.
Translucent bodies
These
are bodies which allow small amount of light falling on them to pass
through e.g oiled paper, some plastic materials and tinted glass.
Transparent bodies
These
are bodies which allow all light to pass through them, e.g glass, pure
water and air. The transmission of light through different bodies can be
investigated by performing other experiment.
Activity 2
Aim: To investigate transmission of light.
Materials
- Sheet of paper
- Sheet of glass
- Tinted glass
- Card board
- Oil paper
Reflection of Light
The Concept of Reflection of Light
Explain the concept of reflection of light
Reflections
are obtained from hard and highly polished surfaces such as mirrors and
sheets of glass than from rougher surfaces, when light falls on the
surface at an inclined plane angle, it is sent back into the air. This
is also the case when light falls on all highly polished metals. If
light falls on a polished surface at an angle 900, it is sent back into the air on the same path.
Difference between Regular and Irregular Reflection of Light
Distinguish regular from irregular reflection of light
Surface can be classified into two types:
- Highly polished surfaces, examples are mirror, polished cooking utensil and silvered iron sheets.
- Rough surface, examples are unpolished wooden table, sheet of paper and cobblestone road.
Application of the Laws of Reflection of Light
Apply the laws of reflection of light
Reflection is guided by some natural laws called “Laws of reflection” the Laws of reflection state that:
- The incident ray, the reflected ray, and the normal at the point of incidence all lies in the same plane.
- The angle of incidence is equal to the angle of reflection.
Image Formed by a Plane Mirror
Describe image formed by a plane mirror
A
clear image is recognised as a result of reflection of the beam
striking the highly polished surfaces. This is called regular
reflection.

Regular reflection.
If
mirrors were replaced by a piece of sheet of paper, there would be no
image to be seen even as a result of diffuse or irregular reflection,
this is shown below.

Irregular or diffuse reflection.
Terms used in reflection
When
light rays strike a surface (e.g a mirror) there are two rays of
importance, namely Incident and Reflected rays, see the figure below:

Reflection of light rays.
- MO is a ray of light falling on a mirror; it is called the incident ray.
- OP is a ray of light leaving the mirror after reflection; it is called the reflected ray. Line NO is perpendicular to the mirror , it is called the normal. ANGLE MON is called the angle of incidence. (i) this is the angle between the incident ray and the normal , angle NOP is called the angle of reflection (t). this is the angle between reflected ray and the normal.
Ndugu mwalimu/mwanafunzi kama unapata changamoto yoyote ile
kuhusu upatikanaji wa NOTES HIZI kwenye website hii, tafadhari wasiliana nasi kwa
simu no/hotline. 0759-104804 Paschal kabonge
barua pepe. paschalkabonge2@gmail.com
au andika maoni yako kwenye sehemu ya comment hapa chini ya post hii.
Kumbuka: mitihani yote inapatikana bureeee kabisa.
Asante.
ELIMU NI HAKI KWA WOTE.





6 Comments
It was really helpful
ReplyDeleteThank you for visiting TLR6, feel free to read our resources and for any inconveniences please contact us. +255759 104804
DeleteThank you too for visiting TLR6
DeleteYou have a best notes how you explain it it's the most I like
ReplyDeleteI really love it
ReplyDeleteThank you TLR6
ReplyDeleteWe have learnt a alot